Big Ideas Math Grade 3 Chapter 13 Classify Two-Dimensional Shapes Answers: Students who are studing grade 3 must know about the geomteric shapes like square, triangle, rectangle and others before learning about two dimensional shapes. You can get the direct link to download Big Ideas Math Book Grade 3 Chapter 13 Classify Two-Dimensional Shapes Answers pdf in the below sections. By learning this chapter, you will be ab;e to define various two dimensional shapes, explain their features and compare one with another and draw shapes.
Big Ideas Math Book Grade 3 Chapter 13 Classify Two-Dimensional Shapes Solutions
Below provided BIM Grade 3 Chapter 13 Classify Two-Dimensional Shapes Answer Key helps the students in easy and quick learning. So, download Big Ideas Answers Grade 3 Chapter 13 Classify Two-Dimensional Shapes PDF for free to kickstartyour preparation. Find the solutions for all BIM 3rd Grade 13th Chapter questions in this article. The various lessons included in Classify Two-Dimensional Shapes Chapter are Identify Sides and Angles of Quadrilaterals, Describe Quadrilaterals, Classify Quadrilaterals, and Draw Quadrilaterals.
After all these lessons, you can see performance task section where you can test your math skills. Improve your performance in the exam byreferring Big Ideas Math Answers Grade 3 Chapter 13 Classify Two-Dimensional Shapes AnswerKey. Scroll down to getthe quick links of all the topics of BIM Grade 3 Chapter 13 Classify Two-Dimensional Shapes.
Lesson 1: Identify Sides and Angles of Quadrilaterals
- Lesson 13.1 Identify Sides and Angles of Quadrilaterals
- Identify Sides and Angles of Quadrilaterals Homework & Practice 13.1
Lesson 2: Describe Quadrilaterals
Lesson 3: Classify Quadrilaterals
Lesson 4: Draw Quadrilaterals
Performance Task
- Classify Two-Dimensional Shapes Performance Task
- Classify Two-Dimensional Shapes Activity
- Classify Two-Dimensional Shapes Chapter Practice
Lesson 13.1 Identify Sides and Angles of Quadrilaterals
Explore and Grow
Sort the Polygon Cards.
 Structure
Structure
Does the sort change if you sort by the number of vertices? Explain.
Think and Grow: Sides and Angles of Quadrilaterals
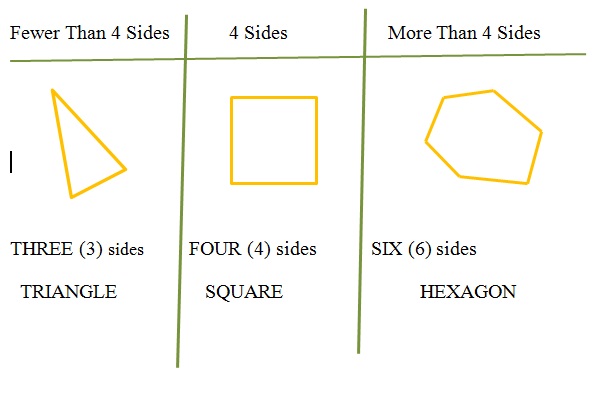
A polygon is a closed, two-dimensional shape with three or more sides.
Answers:
A quadrilateral is a polygon with four sides. Quadrilaterals have four vertices and four angles.
Quadrilaterals can have parallel sides and right angles. Parallel sides are two sides that are always the same distance apart. A right angle is an L-shaped angle.
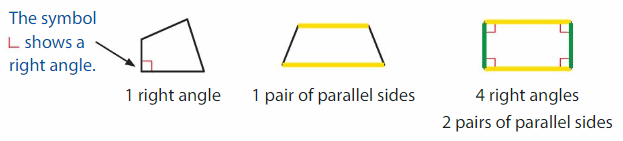
When two straight lines intersect each other at 90˚ or are perpendicular to each other at the intersection, they form the right angle. A right angle is represented by the symbol ∟.
Parallel lines are lines in a plane that are always the same distance apart. Parallel lines never intersect. Parallel lines are represented as ll.
Example
Identify the number of right angles and pairs of parallel sides.
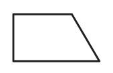
Right angles: ___
Pairs of parallel sides: ___
Answer:
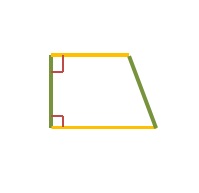
Right angles: 2 (The symbol in pink shows right angles)
Pairs of parallel sides: 1 ( The sides in yellow shows parallel lines)

Right angles: ___
Pairs of parallel sides: ___
Answer:
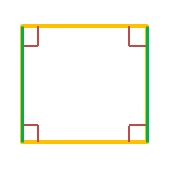
Right angles: 4 (The symbols in pink are right angles).
Pairs of parallel sides: 2 (yellow and green sides are 2 pairs of parallel sides)
Show and Grow
Identify the number of right angles and pairs of parallel sides.
Question 1.
Right angles: ___
Pairs of parallel sides: ___
Answer:
Explanation:
Right Angles : 0
Pairs of parallel sides: 2 (sides in yellow and green are 2 pairs of parallel sides)
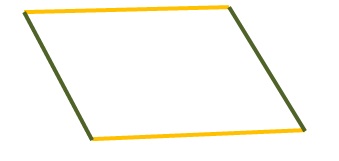
Question 2.

Right angles: ___
Pairs of parallel sides: ___
Answers:
Right angles: 2 (In L shape)
Pairs of parallel sides:0
Apply and Grow: Practice
Identify the number of right angles and pairs of parallel sides.
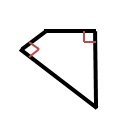
Question 3.
Right angles: ___
Pairs of parallel sides: ___
Answers:
Right angles: 2
Pairs of parallel sides: 1 (1 II 2 as per figure).
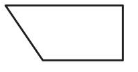
Question 4.

Right angles: ___
Pairs of parallel sides: ___
Answer:
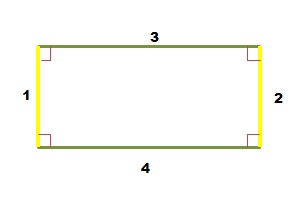
Right angles: 4 (L shape)
Pairs of parallel sides: 2 (sides 1 ll 2 and 3 ll 4 as per above fig).
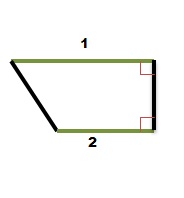
Question 5.
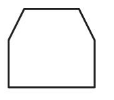
Right angles: ___
Pairs of parallel sides: ___
Answer:
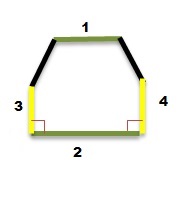
Right angles: 2 (L SHAPE)
Pairs of parallel sides: 2 (sides 1 ll 2 and 3 ll 4).
Question 6.
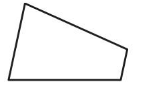
Right angles: ___
Pairs of parallel sides: ___
Answer:

Right angles: 0
Pairs of parallel sides: 0
Question 7.

Right angles: ___
Pairs of parallel sides: ___
Answer:

Right angles: 1
Pairs of parallel sides: 0
Question 8.

Right angles: ___
Pairs of parallel sides: ___
Answer:

Right angles: 1
Pairs of parallel sides: 0
Question 9.
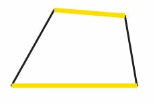
YOU BE THE TEACHER
Your friend says the yellow sides are parallel. Is your friend correct? Explain.
Think and Grow: Modeling Real Life
Use quadrilateral pattern blocks to complete the puzzle.
Answer:
Show and Grow
Question 10.
Use quadrilateral pattern blocks to complete the puzzle.
Answer:
Identify Sides and Angles of Quadrilaterals Homework & Practice
Identify the number of right angles and pairs of parallel sides.
Question 1.
Right angles: ___
Pairs of parallel side: ___
Answer:
Right angles: 0
Pairs of parallel side: 2 (side 1 ll 2 and 3 ll 4)
Question 2.
Right angles: ___
Pairs of parallel side: ___
Answer:
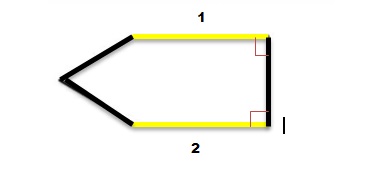
Right angles: 2
Pairs of parallel side: 1 (side 1 ll 2).
Question 3.
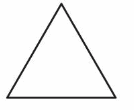
Right angles: ___
Pairs of parallel side: ___
Answer:

Right angles: 0
Pairs of parallel side: 0
Question 4.
Right angles: ___
Pairs of parallel side: ___
Answer:
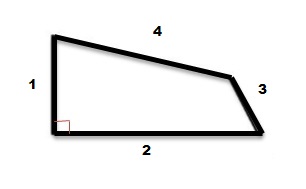
Right angles: 1 (angle between side 1 and 2 )
Pairs of parallel side: 0
Question 5.

Right angles: ___
Pairs of parallel side: ___
Answer:
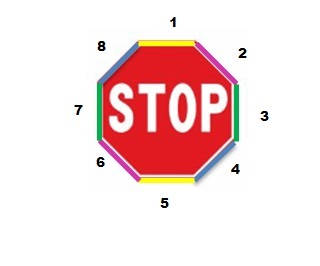
Right angles: 0
Pairs of parallel side: 3 (side 1 ll 5, 2 ll 6, 3 ll 7 and 4 ll 8 as per above figure)
Question 6.

Right angles: ___
Pairs of parallel side: ___
Answer:
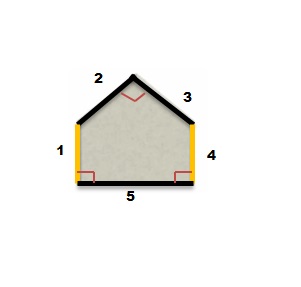
Right angles: 3 ( angles between side 2&3, side 4&5 and 1&5)
Pairs of parallel side: 1 ( sides 1 ll 4).
Question 7.
Reasoning
Can a quadrilateral have exactly three right angles? Explain.
Explanation:
The types of quadrilaterals that have 3 right angles are known as:
1.Squares
2.Rectangles
3.Other shapes where all angles are 90°.
The reason for this is:
All quadrilaterals interior angles must add up to exactly 360°.
So:
= 360−(90+90+90)
= 90°.
And thus, the fourth angle must be 90°.
Thefore, the only quadrilaterals where all angles are 90° are squares and rectangles.
Question 8.
Modeling Real Life
Use pattern blocks to complete the puzzle.
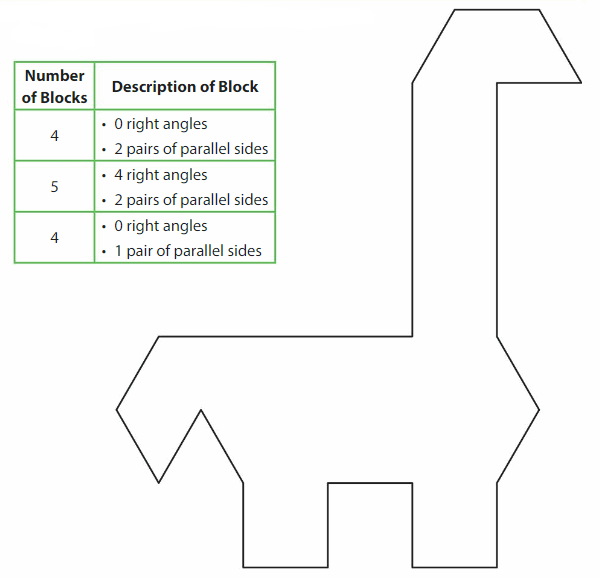
Answer:
Review & Refresh
Question 9.
Newton has 28 cards. Descartes has 24 cards. Newton divides his cards into 4 equal stacks and gives Descartes one stack. How many cards does Descartes have now?
Explanation:
Given:
Cards with Descartes = 24 cards
Cards with Netwon = 28 cards
Cards divided into 4 stacks .
Each stack contains 7 cards
Total cards with Descartes= 24+7= 31 Cards.
Lesson 13.2 Describe Quadrilaterals
Explore and Grow
Sort the Quadrilateral Cards.
Structure
What is another way you can sort the quadrilaterals?
Answer:
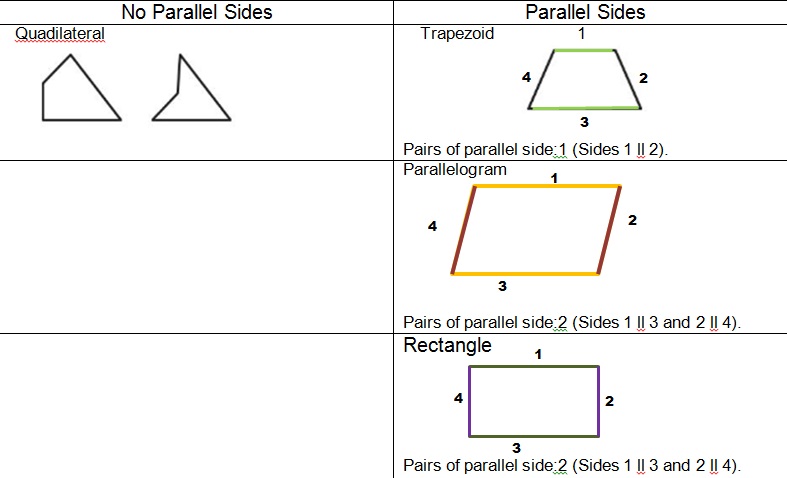
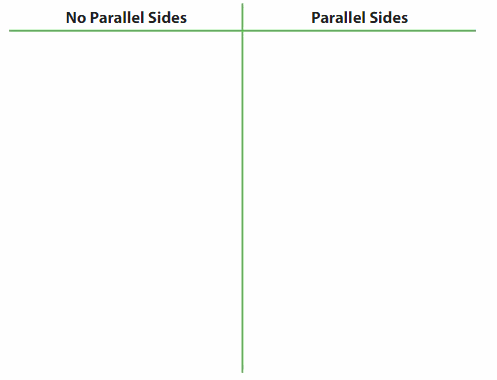
Think and Grow: Idenify Quadrilaterals
You can identify a quadrilateral using its sides and angles. A quadrilateral can have more than one name.
Quadrilateral
4 sides
4 angles
Trapezoid
exactly 1 pair of parallel sides
Parallelogram

2 pairs of parallel sides
Rectangle

2 pairs of parallel sides
4 right angles
Rhombus
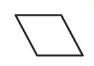
2 pairs of parallel sides
4 equal sides
square

2 pairs of parallel sides
4 equal sides
4 right angles
Example
Circle all of the names for the quadrilateral.
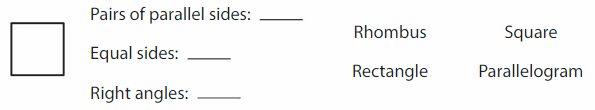
Answer:

Show and Grow
Circle all of the names for the quadrilateral.
Question 1.
Answer:
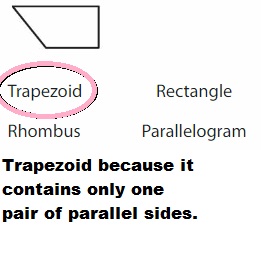
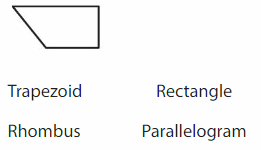
Question 2.

Answer:

Apply and Grow: Practice
Write all of the names for the quadrilateral.
Question 3.
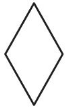
Answer:
Rhombus
2 pairs of parallel sides
4 equal sides
Question 4.
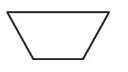
Answer:
Trapezoid – only 1 pair of parallel sides
Name all of the quadrilaterals that can have the given attribute.
Question 5.
2 pairs of parallel sides
Answer:
Square, Rhombus , Rectangle and Parallelogram.
Question 6.
4 right angles
Answer:
Square and Rectangle.
Question 7.
Precision
Is the shape a rhombus? Explain.
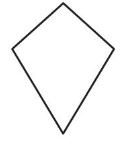
Answer:
No.
Explanation:
Rhombus contains 2 pairs of parallel sides and 4 equal sides but the given figure doesnot have equal sides and parallel sides.
Question 8.
YOU BE THE TEACHER
Your friend not says the shape is a rhombus. Is your friend correct? Explain.

Answer:
Yes .
Explanation:
Rhombus contain 2 pairs of parallel sides and 4 equal sides. but it doesn’t contain 4 right angles. so it is square not rhombus.
Think and Grow: Modeling Real Life
Write all of the names for the red quadrilateral in the painting.
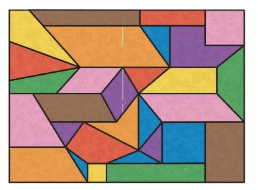
Show and Grow
Use the painting above.
Question 9.
Write all of the names for the blue quadrilateral.
Answer:
1 Square and 4 triangles.
Question 10.
What color is the rhombus that is not a square?
Answer:
Purple.
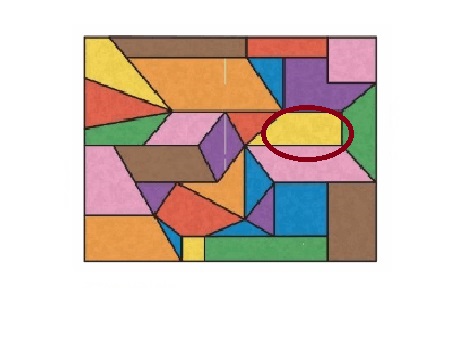
Question 11.
How many trapezoids are in the painting? Circle them.

Answer:
Question 12.
DIG DEEPER!
There are 4 squares and 8 rectangles in a floortile pattern. Find the total number of right angles in the pattern. Explain.
Answer:
one square contain 4 right angles
4 squares = 4×4=16 right angles
one rectangle contain 4 right angles
8 rectangles = 8×4=32 right angles.
Therefore Total Right angles = 16 +32 = 48 angles.
Describe Quadrilaterals Homework & Practice 13.2
Circle all of the names for the quadrilateral.
Question 1.
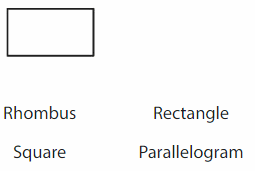
Answer:

Question 2.
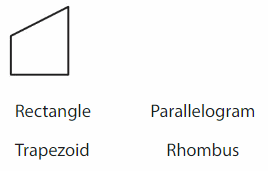
Answer:
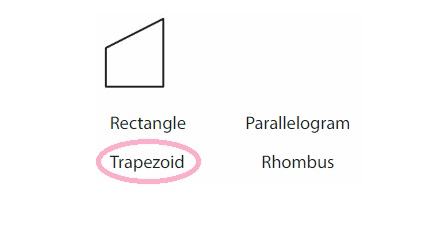
Write all of the names for the quadrilateral.
Question 3.

Answer:
Parallelogram.
Explanation:
It contains 2 pairs of parallel sides.
Question 4.

Answer:
Square .
Explanation:
It contains 2 pairs of parallel sides, 4 equal sides and 4 right angles.
Name all of the quadrilaterals that can have the given attributes.
Question 5.
4 sides and 4 angles
Answer:
Quadilateral.
Question 6.
exactly 1 pair of parallel sides
Answer:
Trapezoid.
Question 7.
Writing
Explain how a trapezoid is different from a parallelogram.
Answer:
A Trapezoid contains only 1 pair of parallel sides where as, parallelogram contains 2 pairs of parallel sides .
Question 8.
Reasoning
Explain why the rectangle shown is not a square.

Answer:
As per the figure only opposite sides are equal but where as square contains all 4 sides equal.
Question 9.
DIG DEEPER!
What is Descartes’s shape?

Answer:
Rectangle.
Question 10.
Which One Doesn’t Belong?
Which not does belong with the other three? Explain.
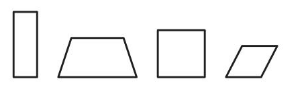
Answer:
Trapezoid as it contains one one parallel side.
Modeling Real Life
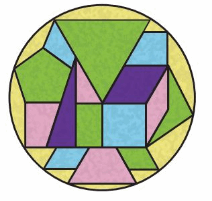
Use the mosaic
Question 11.
Write all of the names for the purple quadrilateral.
Answer:
Triangle and Rhombus
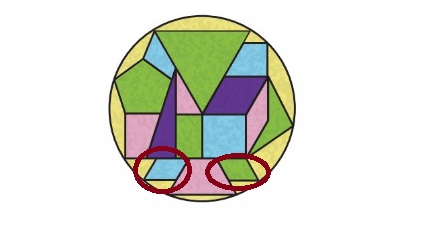
Question 12.
How many parallelograms are in the mosaic? Circle them.
Answer:
Review & Refresh
Question 13.
Find the area of the rectangle.

___6_ × __2_ = ______
Answer:
Area = _________12 sqft____
Explanation:
Area of rectangle is length x Breadth.
Question 14.
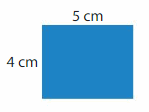
_4___ × 5__ = _____
Area = ____________
Answer:
Area=20 sqcms.
Explanation:
Area of rectangle is length x Breadth.
Lesson 13.3 Classify Quadrilaterals
Explore and Grow
Use each description to model a quadrilateral on your geoboard. Draw each quadrilateral.
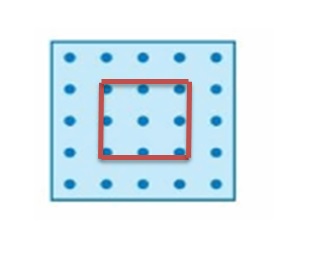
Two pairs of parallel sides

Answer:
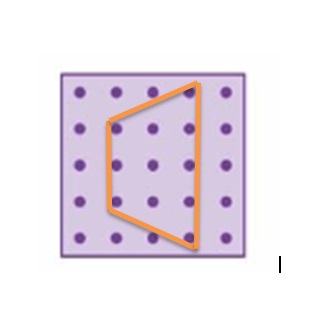
Exactly one pair of parallel sides

Answer:
Four right angles that do not form a square

Answer:

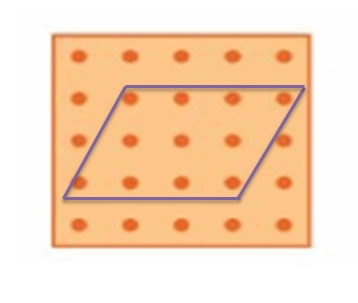
Two pairs of parallel sides and no right angles

Answer:
Structure
Compare your quadrilaterals to your partner’s. Are your quadrilaterals the same? Are you both correct? Explain.
Answer:
Different Answer for 1 condition because Two pairs of parallel sides are rectangle,square,rhombus and parallelogram.
Same Answers for 2,3 and 4 conditions.
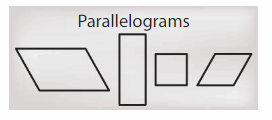
Think and Grow:
How are the parallelograms and rhombuses alike? How are they different?

Ways they are alike:
Each has ___ sides.
Each has ___ angles.
Each has ___ pairs of parallel sides.
Answer:
A parallelogram is a rhombus if the diagonals are perpendicular.
Ways they are alike:
Each has __4_ sides.
Each has __2_ angles.
Each has __2_ pairs of parallel sides.
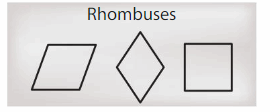
Ways they are different:
Rhombus always have __4__ equal sides.
What names can you use to classify all parallelograms and rhombuses?
Answer:
Squares and Rectangles.
A rectangle has two sets of parallel sides, so it is a parallelogram.
A rhombus has four congruent sides and a square has four congruent sides and angles. Therefore, a rhombus is a square when it has congruent angles.
Show and Grow
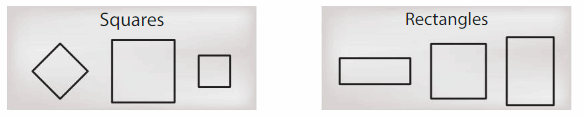
Question 1.
How are squares and rectangles alike? How are they different?
Answer:
Squares and rectangles both are a type of quadrilateral, both have their four interior angles equal to 90 degrees and opposite sides parallel to each other.
The difference between square and rectangle is that a square has all its sides equal whereas a rectangle has its opposite sides equal.
Question 2.
What names can you use to classify all squares and rectangles?
Answer:
Parallelogram because A Rectangle is a Parallelogram with all right angles. A Square is a Rectangle with adjacent sides equal.
Question 3.
Draw a quadrilateral that is not a square or a rectangle. Explain.
Answer:
Explanation:
Trapezoids contain only 1 pair of parallel sides.
Apply and Grow: Practice
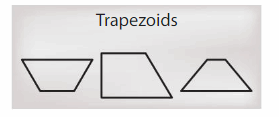
Question 4.
How are trapezoids and parallelograms alike? How are they different?
Answer:
A trapezoid has one pair of parallel sides and a parallelogram has two pairs of parallel sides.
A trapezoid can be called a parallelogram when it has more than one pair of parallel sides.
Question 5.
What name can you use to classify all trapezoids and parallelograms?

Question 6.
How are rhombuses and squares alike? How are they different?
Answer:
Rhombuses and Square are alike as they contain 2 pairs of parallel sides and 4 equal sides. Different as Square have 4 right angles, but rhombus does not.
Question 7.
What names can you use to classify all rhombuses and squares?
Answer:
Parallelogram.
Question 8.
Draw a quadrilateral that is a rhombus but not a square.
Answer:
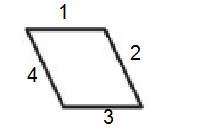
Question 9.
Draw a quadrilateral that is not a rhombus or a square. Explain.
Answer:
Think and Grow: Modeling Real Life
Sort the road signs into two groups by shape. What is alike and what is different between the two groups? What name can you use to classify all of the road sign shapes?

Answer:


Explanation:
Rectangles contain 2 pairs of parallel sides but where as , Trapezoid contain only 1 pair of parallel sides .
Show and Grow
Question 10.
Sort the road signs into two groups by shape. What is alike and what is different between the two groups? What names can you use to classify all of the road sign shapes?

Answer:

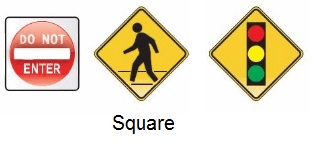
Explanation:
Square and Rectangles are alike as they contain 2 pairs of parallel sides and
4 right angles. they are different as square contain all sides equal but rectangle contain only opposite sides equal.
Classify Quadrilaterals Homework & Practice 13.3
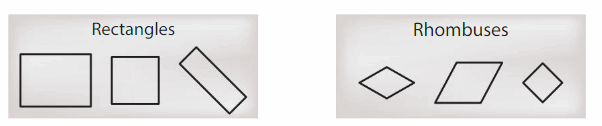
Question 1.
How are rectangles and rhombuses alike? How are they different?
Answer:
Rectangle and Rhombus are alike because both contain 2 pairs of parallel lines. they are different because rectangle contain 4 right angles and opposite sides equal.Rhombus contain all sides equal with 0 right angles.
Question 2.
What names can you use to classify all rectangles and rhombuses?
Answer:
Parallelogram.
Question 3.
DIG DEEPER!
Your friend says a shape is a rectangle. Newton says the same shape is a rhombus, and Descartes says it is a square. Can they all be correct? Explain.
Answer:
In rhombus,Square and Rectangle all the opposite sides are equal.
Question 4.
YOU BE THE TEACHER
Is Newton correct? Explain.

Answer:
Trapezoids have only one pair of parallel sides; parallelograms have two pairs of parallel sides. A trapezoid can never be a parallelogram.
Question 5.
Modeling Real Life
Sort the earrings into two groups by shape. What is alike and what is different between the two groups? What name can you use to classify all of the earring shapes?
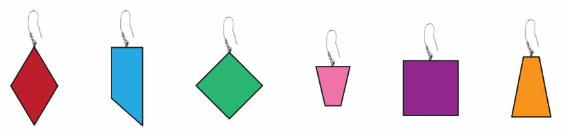
Answer:
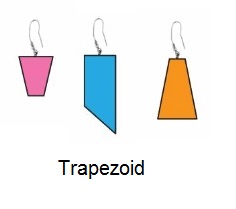

Explanation:
Trapezoid contain only 1 pair of parallel sides .Square contains 2 pairs of parallel sides .
Review & Refresh
Question 6.
Use the number line to find an equivalent fraction.
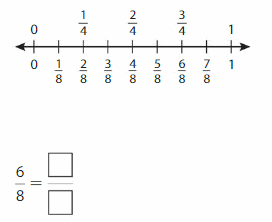
Answer:
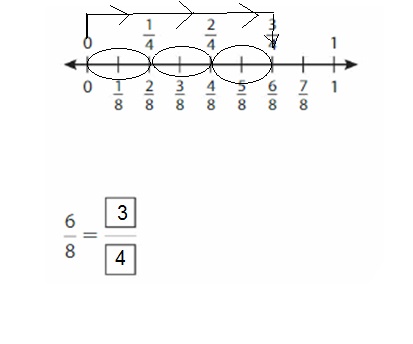
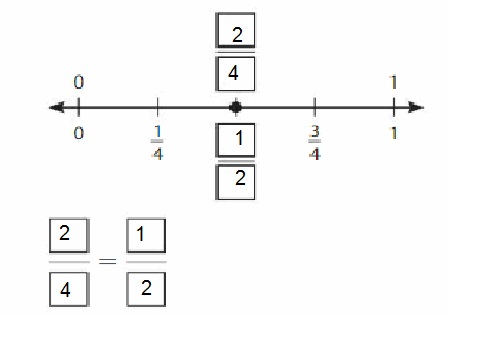
Question 7.
Write two fractions that name the point shown.
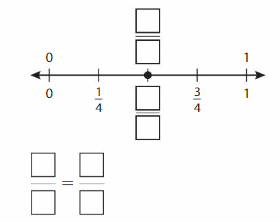
Answer:
Lesson 13.4 Draw Quadrilaterals
Explore and Grow
Model each quadrilateral on your geoboard. Move one vertex of each quadrilateral to create a new quadrilateral. Draw each new quadrilateral.
Answer:
Structure
Create your own quadrilateral. Move one vertex to create a new quadrilateral. Draw your quadrilaterals. Name each quadrilateral

Answer:

Think and Grow: Draw Quadrilaterals
Example
Draw a quadrilateral that has four right angles. Name the quadrilateral.
Answer:
Square.

Example
Below are three rhombuses. Draw a quadrilateral not that is a rhombus. Explain why it is not a rhombus.
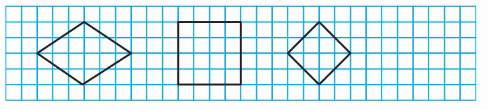
The quadrilateral is not a rhombus because _______
______________________________________.
Answer:
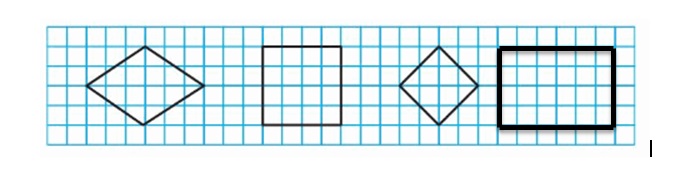
Explanation:
The quadrilateral is not a rhombus because rectangle contains only opposite sides equal .
Show and Grow
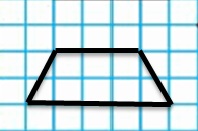
Question 1.
Draw a quadrilateral that has exactly one pair of parallel sides. Name the quadrilateral.

Answer:
Trapezoid.
Question 2.
Draw a quadrilateral that is not a square. Explain why it is not a square.

Answer:
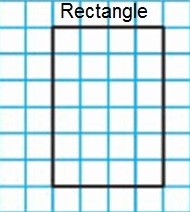
Explanation:
Square contains 2 pairs of parallel sides , 4 equal sides and 4 right angles.
Rectangle contains 2 pairs of parallel sides and 4 right angles .
Apply and Grow: Practice
Name the group of quadrilaterals. Then draw a quadrilateral that does not belong in the group. Explain why it does not belong.
Question 3.
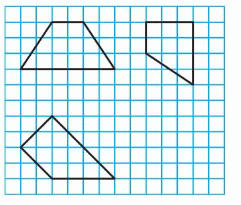
Answer:
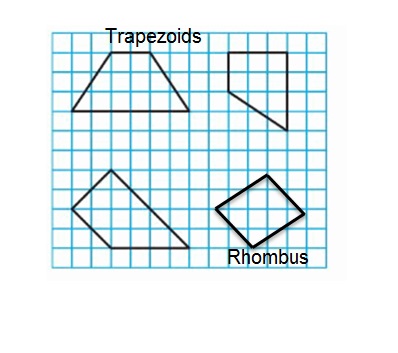
Explanation:
Trapezoid contains only 1 pair of parallel side but whereas rhombus contain 2 pairs of parallel sides .
Question 4.
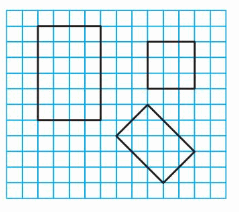
Answer:
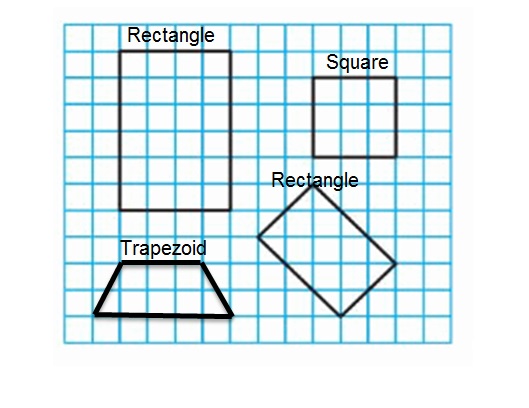
Explanation:
Rectangle and Square contains 2 pairs of parallel sides but whereas Trapezoid contains only 1 pair of parallel sides .
Question 5.
Precision
Circle the quadrilaterals that are not rhombuses.
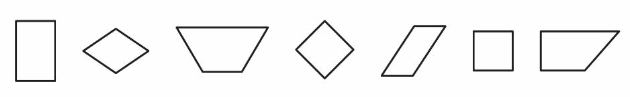
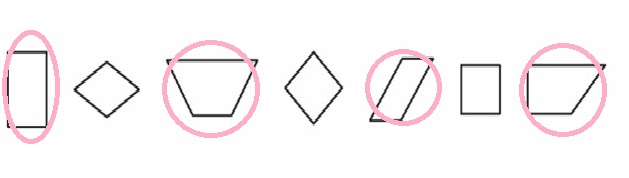
Answer:
Question 6.
YOU BE THE TEACHER
Your friend draws the shape and says it is a parallelogram because it has two pairs of parallel sides. Is your friend correct? Explain.

Answer:
It is a pentagon as it contains 5 sides, whereas parallelogram contain only 4 sides.
Question 7.
DIG DEEPER!
Draw the quadrilateral with two pairs of parallel sides. One side is given.
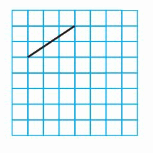
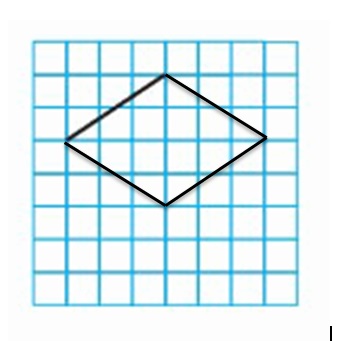
Answer:
Think and Grow: Modeling Real Life
A helicopter travels to various Colorado cities. Draw to show a route that forms a parallelogram. Write the names of the cities you use.
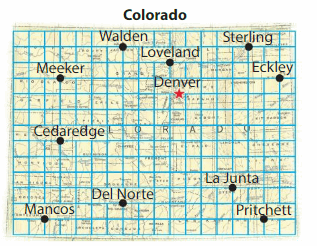
Answer:

Sterling, eckley, pritchett and La junta forms parallelogram .
Show and Grow
Question 8.
Use the map above. Drawtoshow a route that forms a trapezoid. Write the names of the cities you use.
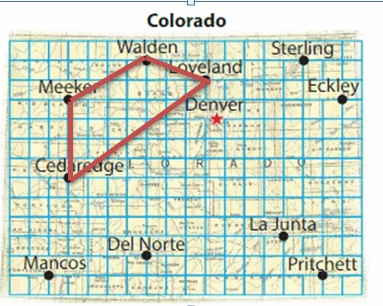
Meeker,walden,loveland and cedaredge.
Question 9.
You have four markers of equal length. Name all of the quadrilaterals you can make using the markers as sides.

Answer:
Rhombus and Square .
Question 10.
DIG DEEPER!
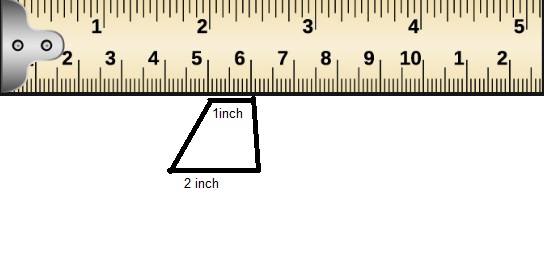
Use a ruler to draw a trapezoid for each description.
- one side length of 1 inch
- one side length of 1 inch
- one side length of 2 inches
- one side length of 2 inches
- two right angles
- no right angles
Answer:
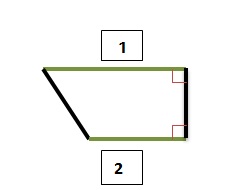
Trapezoid with 2 right angles
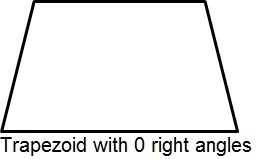
Draw Quadrilaterals Homework & Practice 13.4
Question 1.
Draw a quadrilateral that has two pairs of parallel sides. Name the quadrilateral.

Answer:
Question 2.
Draw a quadrilateral that is not a rhombus.

Answer:
Question 3.
Name the group of quadrilaterals. Then draw a quadrilateral that does not belong in the group. Explain why it does not belong.
Answer:
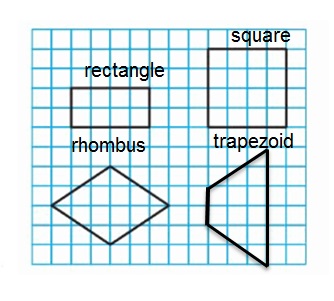
Explanation:
Square, Rhombus and Rectangle contains 2 pairs of parallel sides. where Trapezoid contains only 1 pair of parallel side .
Question 4.
Precision
Circle the quadrilaterals that are not trapezoids.
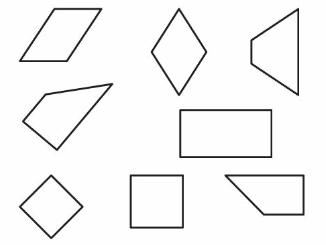
Answer:
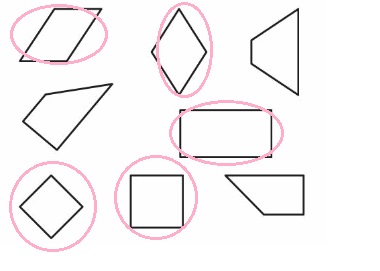
Question 5.
Modeling Real Life
A bus tour wants to travel to various locations. Draw to show a route that forms a rectangle. Write the names of the location drop-off points you use.
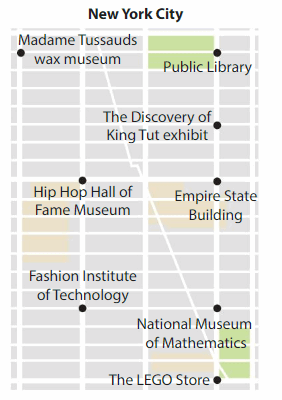
Answer:
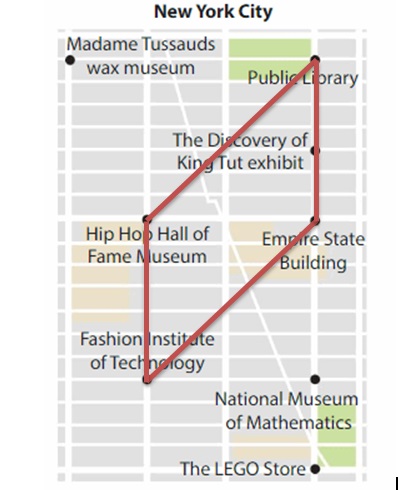
The Drop off point is The Discovery of king Tut exhibit .
Review & Refresh
Question 6.
Tell whether the shape shows equal parts or unequal parts. If the shape shows equal parts, then name them

_____ parts _____
Answer:
11 square parts – equal parts .
8 rectangle parts – unequal parts .
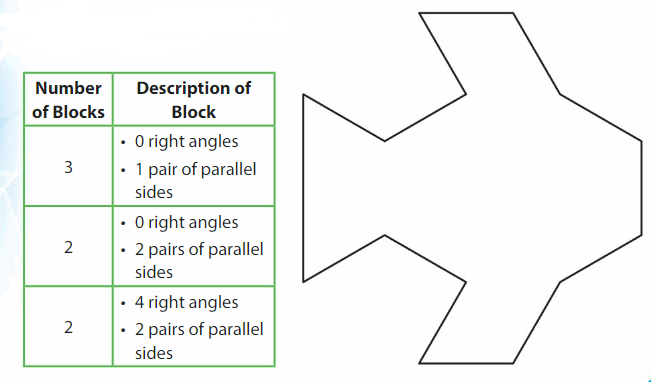
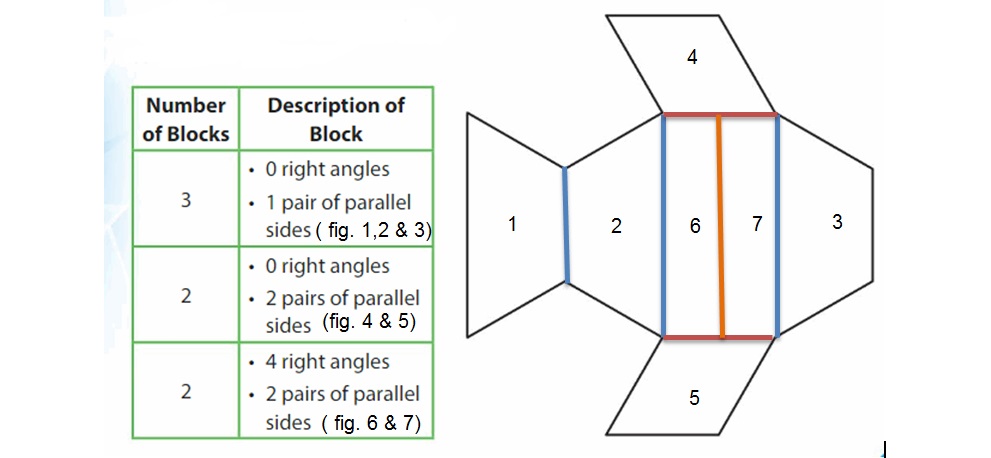
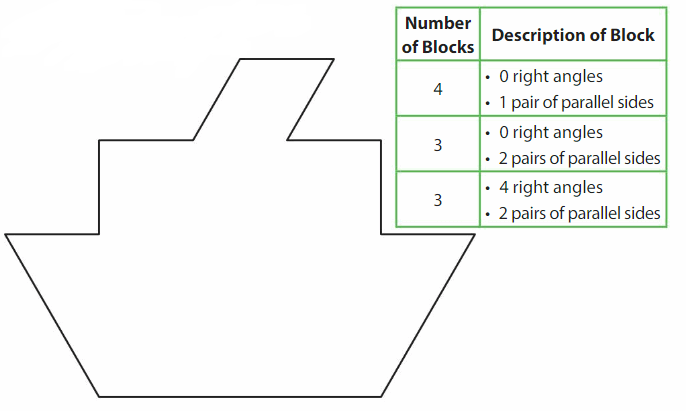
Classify Two-Dimensional Shapes Performance Task
Your class is learning about fossils.
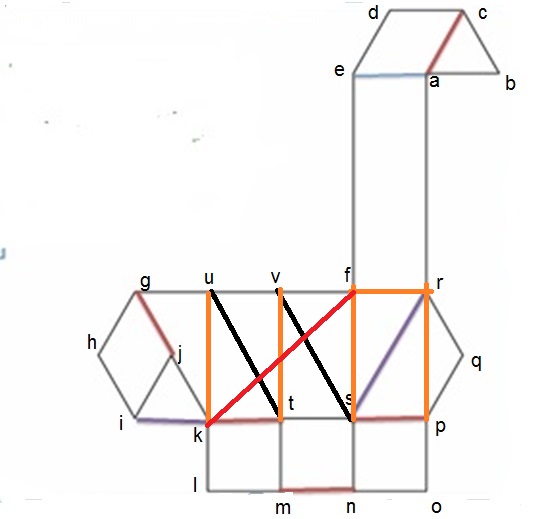
Question 1.
Your teacher wants to create cards using the fossils below. Use each polygon description to write the fossil name on the correct card.
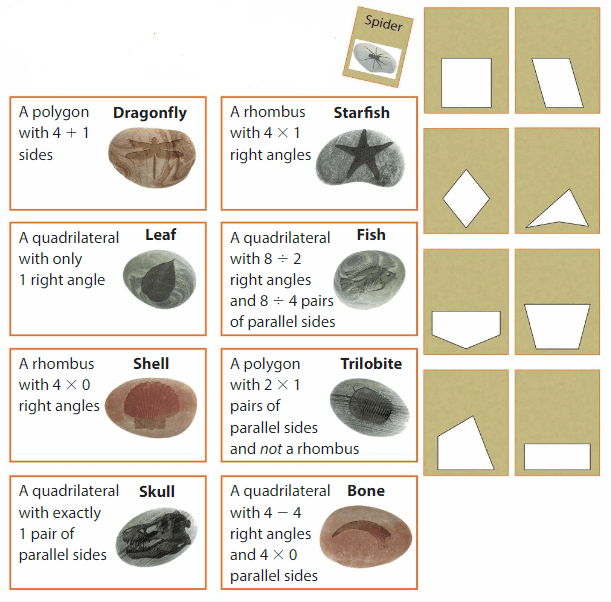
Answer: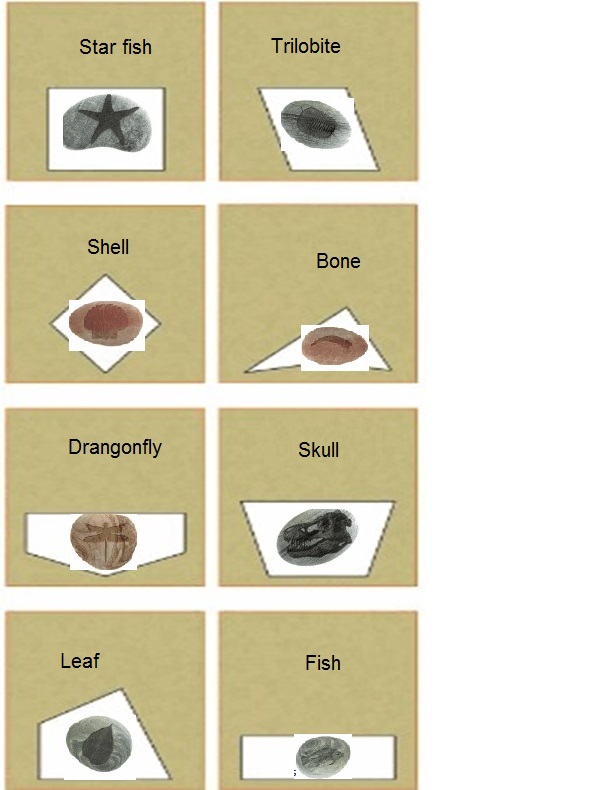
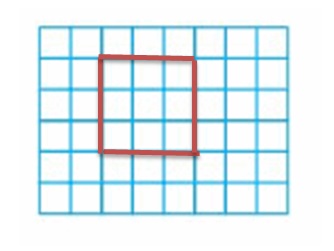
Question 2.
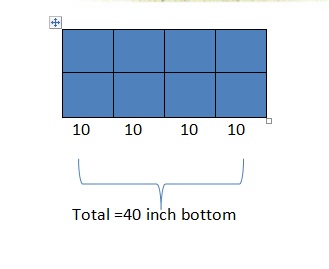
Your teacher uses the cards and a sandbox to create an archaeological dig site. Your teacher lays a grid with eight grid squares over the top of the sandbox. Each square has 10-inch side lengths. How many square inches is the bottom of the sandbox?
Answer:
Classify Two-Dimensional Shapes Activity
Identify That Quadrilateral!
Directions:
1. Players take turns rolling a die.
2. On your turn, move your piece the number of spaces shown on the die.
3. Cover a space on the board that describes the shape where you landed.
4. If there are no spaces that match your shape, then you lose your turn.
5. The first player to cover six spaces wins!
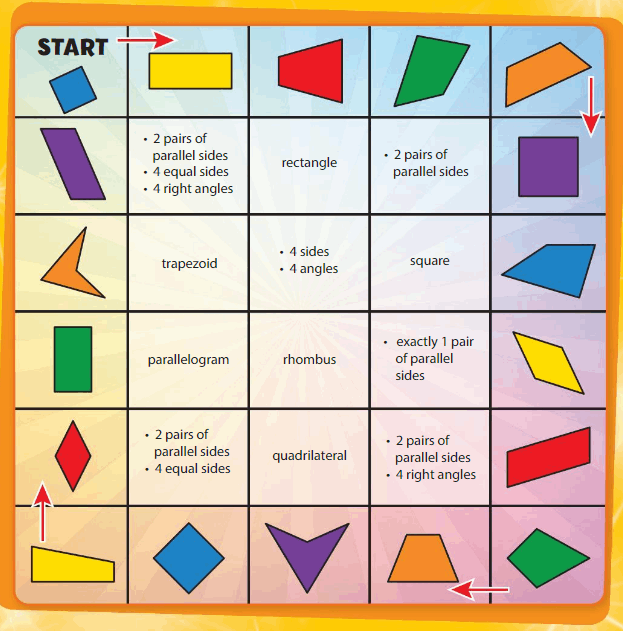
Answer:
Classify Two-Dimensional Shapes Chapter Practice
13.1 Identify Sides and Angles of Quadrilaterals
Identify the number of right angles and pairs of parallel sides.
Question 1.
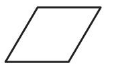
Right angles: ____
Pairs of parallel sides: ___
Answer:
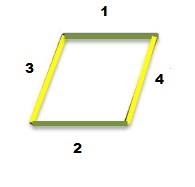
Right angles: __0__
Pairs of parallel sides: __2_( sides 1 ll 2 and 3 ll 4 ) .
Question 2.
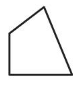
Right angles: ____
Pairs of parallel sides: ___
Answer:
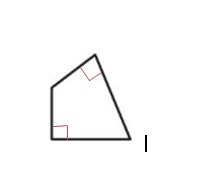
Right angles: 2
Pairs of parallel sides: 0
Question 3.
YOU BE THE TEACHER
Your friend says the yellow sides are parallel. Is your friend correct? Explain.

Answer:
Yes.
Explanation:
It is a Trapezoid it contains only 1 pair of parallel sides.
13.2 Describe Quadrilaterals
Question 4.
Write all of the names for the quadrilateral.

Answer:
Rectangle
Question 5.
Name all of the quadrilaterals that can have no right angles.
Answer:
Trapezoid, Rhombus and Parallelogram.
13.3 Classify Quadrilaterals

Question 6.
How are squares and trapezoids alike? How are they different?
Answer:
Both are quadrilaterals, so both of them have four sides. The square has two pairs of parallel sides, while the trapezoid only has one pair of parallel sides. So, we can say that both have one pair of parallel sides in common.
squares have all sides equal . but whereas trapezoid sides are different.
squares have 4 right angles but whereas trapezoid have different angles.
Question 7.
What name can you use to classify all squares and trapezoids?
Answer:
Quadilaterals and Parallelogram.
13.4 Draw Quadrilaterals
Question 8.
Draw a quadrilateral that has four right angles. Name the quadrilateral.

Answer:
Square.
Question 9.
Modeling Real Life
A helicopter travels to various locations in Wyoming. Draw to show a route that forms a square. Write the names of the locations you use.
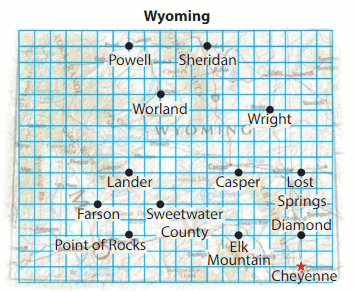
Answer:
lander, farson, points of rocks and sweetwater.
Big Ideas Math Answers 3rd Grade Chapter 13 Classify Two-Dimensional Shapes
I hope that the details prevailed in this page regarding Big Ideas Math Book Grade 3 Chapter 13 Classify Two-Dimensional Shapes Answer Key is useful for all the students and also for teachers. Make use of the answers to secure best grades in the exams. Stay in touch with oursite to get the latest solutions for other Big Ideas Grade 3 Chapters.