Big Ideas Math Book 3rd Grade Answer Key Chapter 1 Understand Multiplication and Division: Get the solutions for all the questions in BIM Grade 3 Chapter 1 Understand Multiplication and Division Textbook. This answer key is useful for the students while preparing for the exam or practice test. In the Big Ideas Math 3rd Grade 1st Chapter Solutions PDF, students can see the detailed explanation for each problem that helps them to understand the concept easily.
Big Ideas Math Book Grade 3 Answer Key Chapter 1 Understand Multiplication and Division
Download Big Ideas Math 3rd Grade 1st Chapter Understand Multiplication and Division Answer Key PDF for free of cost. The different lessons in Grade 3 Chapter 1 are Use Equal Groups to Multiply, Use Number Lines to Multiply, Use Arrays to Multiply, Multiply in Any Order, Divide: Size of Equal Groups, Divide: Number of Equal Groups, and Use Number Lines to Divide.
Here, you can get the different methods of solving multiplication and division problems. Solve all the questions from Big Ideas Math Answers Grade 3 Chapter 1 Understand Multiplication and Division. Tap on the links mentioned below to get help while solving multiplication and division problems.
Lesson 1 Use Equal Groups to Multiply
Lesson 2 Use Number Lines to Multiply
Lesson 3 Use Arrays to Multiply
Lesson 4 Multiply in Any Order
Lesson 5 Divide: Size of Equal Groups
Lesson 6 Divide: Number of Equal Groups
Lesson 7 Use Number Lines to Divide
Performance Task
- Understand Multiplication and Division Performance Task
- Understand Multiplication and Division Activity
- Understand Multiplication and Division Chapter practice
Lesson 1.1 Use Equal Groups to Multiply
Explore and Grow
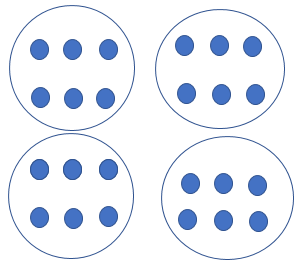
Question 1.
Put 24 counters into equal groups. Draw to show your groups.
Answer:
24 counters in four equal groups
Explanation:
To keep 24 counters into an equal group, we will find the factors of 24 and then we can put 24 counters into equal groups. The factors of 24 are 2,3,4,6,8,12. Now we can pick any of the numbers from the factors of 24 and then put these 24 counters in equal groups. Let’s take four equal groups and arrange 24 counters in four equal groups as shown in the below image.
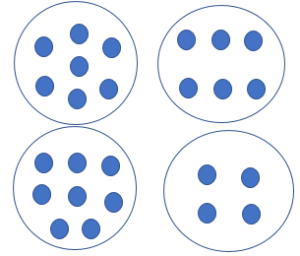
Question 2.
Put 24 counters into a different number of equal groups. Draw to show your groups.
Answer:
7 counters in the first group, 8 counters in the second group, 6 counters in the third group, and 4 counters in the fourth group.
Explanation:
In this, we will take some number of groups and in that, we will place some different number of counters in that group. In the given image, we will take four groups, and we will place 7 counters in the first group, 8 counters in the second group, 6 counters in the third group, and 4 counters in the fourth group.
Question 3.
Structure
Compare your models. How are the models the same? How are they different?
Answer:
In the first model, we can see that there is an equal number of counters in each group, and in the second model, we can see the different number of counters in each group. They are the same with an equal number of groups.
Show and Grow
Use the model to complete the statements.
Question 1.
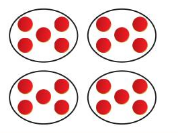
____ groups of ____
____ + ____ +____ + ____ = ____
____ × ____ = ____
Answer:
4 groups of 5,
5+5+5+5= 20,
4×5= 20.
Explanation:
In the above image, we can see 4 groups and in each group, 5 counters are there, and the product is 4×5= 20. And we can also write by adding 5+5+5+5= 20. There are 4 groups of 5.
Question 2.
____ groups of ____
____ + ____ = ____
____ × ____ = ____
Answer:
2 groups of 6,
6+6= 12,
2×6= 12.
Explanation:
As we can see there are 2 groups of 6 which are two groups with the number 6 in the box. So the product of the two groups is 2×6= 12 and the sum of the two groups is 6+6= 12.
Apply and Grow: Practice
Question 3.
Use the model to complete the statements.
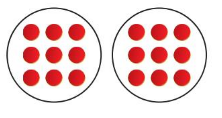
____ groups of ____
____ + ____ = ____
____ × ____ = ____
Answer:
2 groups of 9
9+9= 18,
2×9= 18.
Explanation:
In the above image, we can see 2 groups with 9 counters in each group, and the product is 2×9= 18. By adding these two groups we will get 18, which is 9+9= 18.
Draw equal groups. Then complete the equations.
Question 4.
4 groups of 2
____ + ____ + ____ + ____ =____
____ × ____ = ____
Answer:
4 groups of 2
2+2+2+2= 8,
4×2= 8.
Explanation:
In the above image, we can see 4 groups with 2 counters in each group, and the product is 4×2= 8. By adding these four groups we will get 8, which is 2+2+2+2= 18.
Question 5.
3 groups of 5
____ + ____ + ____ = ____
____ × ____ = ____
Answer:
3 groups of 5,
5+5+5= 15,
3×5= 15.
Explanation:
In the above image, we can see 3 groups with 5 counters in each group, and the product is 3×5= 15. By adding these three groups we will get 15, which is 5+5+5= 15.
Write the addition equation as a multiplication equation.
Question 6.
8 + 8 + 8 = 24
Answer:
3×8= 24.
Explanation:
To write the addition equation as a multiplication equation in the above we can see 3 times 8 which can be written as 3×8= 24.
Question 7.
7 + 7 + 7 + 7 + 7 = 35
Answer:
5×7= 35.
Explanation:
To write the addition equation as a multiplication equation in the above we can see 5 times 7 which can be written as 5×7= 35.
Question 8.
You Be The Teacher
Newton says he circled 3 groups of 4 counters. Is he correct? Explain.
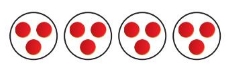
Answer:
No, Newton is not correct.
Explanation:
In the above image, we can see 4 groups of 3 counters. So Newton is not correct.
Question 9.
DIG DEEPER!
You wash 5 cars. How many times do you wash?

Answer: 20 tires.
Explanation:
As the car has 4 tires and there are 5 cars to wash, so there are 5 groups of 4. The number of tires to wash is
5×4= 20. So there are 20 tires to wash.
Think and Grow: Modeling Real Life
Question 1.
You buy 6 packs of 10 trading cards. How many cards do you buy in all?
Complete the statement: ______ groups of ________
Repeated addition equation:
Multiplication equation:
You buy _____ cards in all.
Answer:
6 groups of 10
6×10= 60.
Explanation:
As there are 6 packs of 10 trading cards, which means 6 groups of 10. So 6×10= 60 cards can we buy in all.
Show and Grow
Question 10.
You buy 8 packs of 4 highlighters. How many highlighters do you buy in all?

Answer:
8 groups of 4
8×4= 32
Explanation:
As there 8 packs of 4 highlighters, which means 8 groups of 4. The number of highlighters bought is 8×4= 32.
Question 11.
DIG DEEPER!
You make 5 bracelets. Each of your bracelets has 3 beads. Your friend makes 6 bracelets. Each of your friend’s bracelets has 2 beads. How many beads do you and your friend use in all?

Answer:
27 beads.
Explanation:
In this query, we can see 5 bracelets have 3 beads which are 5×3= 15, and a friend makes 6 bracelets that have 2 beads which are 6×2= 12. So the total number of beads used in all is 15+12= 27 beads.
Use Equal Groups to Multiply Homework & Practice 1.1
Question 1.
Use the model to complete the statements.
___ groups of ____
___ + ___ + ___ = ___
___ × ___ = ___
Answer:
3 groups of 4,
4+4+4= 12,
4×3= 12.
Explanation:
We can see in the above image there are 3 groups with 4 counter in each group, which means 3 groups of 4.
The sum is 4+4+4= 12 and the product is 4×3= 12.
Draw equal groups. Then complete the equations.
Question 2.
2 groups of 8
___ + ___ = ___
___ × ___ = ___
Answer:
8+8= 16
2×8= 16.
Explanation:
2 groups of 8 means, there are 2 groups with 8 counters in each group. So the sum is 8+8= 16 and the product is 2×8= 16.
Question 3.
5 groups of 3
___ + ___ + ___ + ___ + ___ = ___
___ × ___ = ___
Answer:
3+3+3+3+3= 15,
5×3= 15.
Explanation:
5 groups of 3 means, there are 5 groups with 3 counters in each group. So the sum is 3+3+3+3+3= 15, and the product is 5×3= 15.
Write the addition equation as a multiplication equation.
Question 4.
3 + 3 + 3 + 3 + 3 + 3 = 18
Answer:
6×3= 18.
Explanation:
To write the addition equation as a multiplication equation in the above we can see 6 times 3, which can be written as 6×3= 18.
Question 5.
2 + 2 + 2 + 2 + 2 + 2 + 2 = 14
Answer:
7×2= 14.
Explanation:
To write the addition equation as a multiplication equation in the above we can see 7 times 2, which can be written as 7×2= 14.
Question 6.
DIG DEEPER!
You have 16 action figures. Can you put an equal number of figures on 3 shelves? Explain
Answer:
No, we cannot put an equal number of figures on 3 shelves.
Explanation:
As given that we have 16 action figures and asked to determine whether it is possible to an equal number of figures on 3 shelves. As factors of 16 are 1,2,4,8,16 as 16 is not divisible by 3, so we cannot put an equal number of figures on 3 shelves.
Question 7.
Which One Doesn’tBelong?
Which one does not belong with the other two?
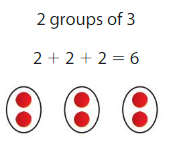
Answer:
2 groups of 3 don’t belong with the other two.
Explanation:
As 2 groups of 3 means in 2 groups, there will be 3 counters, which does not belong to the other two. Because in the above image we can see 3 groups of 2, which means 3 groups with 2 counters in each group which is equal to 2+2+2= 6.
Question 8.
Modeling Real Life
You make 7 gift bags for your friends. Each gift bag has 3 pom-pom pets. How many pom-pom pets are there in all?
Answer:
21 pom-pom pets.
Explanation:
As there are 7 gift bags and each gift bag has 3 pom-pom pets, so the number of pom-pom pets are 7×3= 21.
Question 9.
DIG DEEPER
Newton has 2 stacks of 5 books. Descartes has 3 stacks of 4 books. How many books do they have in all?

Answer:
22 books.
Explanation:
As Newton has 2 stacks of 5 books, which is 2×5= 10 books and Descartes has 3 stacks of 4 books which is 3×4= 12 books. So the number of books is 10+12= 22books.
Review & Refresh
Question 10.
50 + 30 = ____
Answer:
80
Explanation:
By adding 50+30 we will get 80.
Question 11.
27 + 40 = ______
Answer:
67
Explanation:
By adding 27+40 we will get 67.
Question 12.
19 + 20 = _____
Answer:
39
Explanation:
By adding 19+20 we will get 39.
Lesson 1.2 Use Number Lines to Multiply
Explore and Grow
Question 1.
Find the sums. Use each sum as the missing addend in the next equation. Model the problems on the number line.
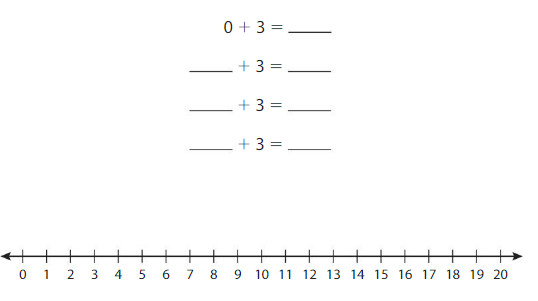
Answer:
0+3= 3,
3+3= 6,
6+3= 9,
9+3= 12.
Explanation:
First, we must begin at 0 and then skip the count by 3’s then make jumps until you get 12.
So 3 x 4 = 12.
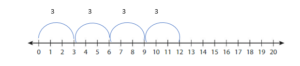
Reasoning
How can you use a number line to help you find 4 × 3?
Answer:
4×3= 12.
Explanation:
To find 4×3 using the number line, we will make a jump from 0 to 4 for 3 times.
Think and Grow: Multiplication and Number Lines
Example Find 3 × 4

3 × 4 means 3 groups of 4.
Number of jumps: _____
Size of each jump: ______
Start at 0. Skip count by 4s three times.
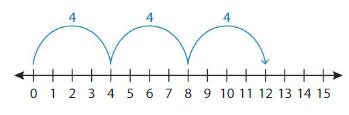
3 × 4 ____
Answer:
3 × 4 = 12
Number of jumps: 3
Size of each jump: 4.
Explanation:
To represent the given value in the number line, we will start from 0 and skip the count by 4 three times. So the number of jumps is 3 and the size of each jump is 4.
Show and Grow
Question 1.
Find 2 × 4
Number of jumps: _____
Size of each jump: ______
2 × 4 = _____
Answer:
2 × 4 = 8,
Number of jumps: 2
Size of each jump: 4
Explanation:
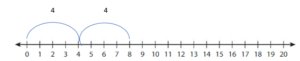
To find the product of 2×4 using the number line, we will start from zero and then skip the count by 4s two times. So the number of jumps is 2 and the size of each jump is 4.
Question 2.
Fing 6 × 3
Number of jumps: _____
Size of each jump: ______
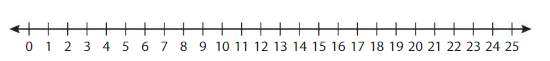
6 × 3 = _____
Answer:
6 × 3= 18
Number of jumps: 6
Size of each jump: 3
Explanation:
To find the product of 6 × 3 using the number line, we will start from zero and then skip the count by 3s six times. So the number of jumps is 6 and the size of each jump is 3.
Apply and Grow: Practice
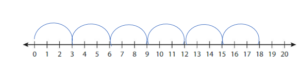
Question 3.
Find 3 × 5
Number of jumps: _____
Size of each jump: ______
3 × 5 = _____
Answer:
3 × 5= 15
Number of jumps: 3
Size of each jump: 5
Explanation:
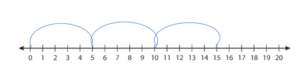
To find the product of 3 × 5 using the number line, we will start from zero and then skip the count by 5s three times. So the number of jumps is 3 and the size of each jump is 5.
Question 4.
Fing 5 × 4
Number of jumps: _____
Size of each jump: ______
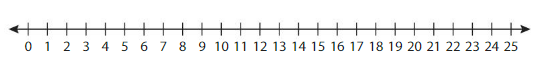
5 × 4 = _____
Answer:
5 × 4= 20
Number of jumps: 5
Size of each jump: 4
Explanation:
To find the product of 5 × 4 using the number line, we will start from zero and then skip the count by 4s five times. So the number of jumps is 5 and the size of each jump is 4.
Question 5.
Find 3 × 8
Number of jumps: _____
Size of each jump: ______
Answer:
3 × 8= 24
Number of jumps: 8
Size of each jump: 3
Explanation:

To find the product of 3 × 8 using the number line, we will start from zero and then skip the count by 3s eight times. So the number of jumps is 8 and the size of each jump is 3.
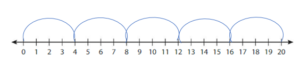
Question 6.
Structure
Draw jumps to show 4 groups of 6 and 6 groups of 4. Think: How are they the same? How are they different?
Answer:
4 groups of 6
4×6= 24
Number of jumps: 6
Size of each jump: 4
6 groups of 4
6×4= 24
Number of jumps: 4
Size of each jump: 6
The product of 4 groups of 6 and 6 groups of 4 is 24 this is the same in 4 groups of 6 and 6 groups of 4. The number of jumps and the size of the jumps is different.
Explanation:
To find the product of 4 × 6 using the number line, we will start from zero and then skip the count by 4s six times. So the number of jumps is 6 and the size of each jump is 4.
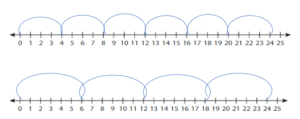
To find the product of 6 × 4 using the number line, we will start from zero and then skip the count by 6s four times. So the number of jumps is 4 and the size of each jump is 6.
Think and Grow: Modeling Real Life
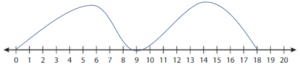
A group of lions is called a pride. There are 2 prides in a savanna. Each pride has 9 lions. How many lions are there in all?

Model:
There are ____ lions in all.
Answer:
2×9= 18 lions.
Explanation:
As there are 2 prides in the savanna and each pride has 9 lions, the total number of lions is 2×9= 18 lions.
To represent this in the number line, we will start from 0 then skip the count by 9 twice. So the number of jumps is 2 and the size of the jump is 9.
Show and Grow
Question 7.
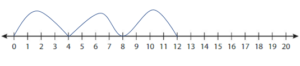
There are 3 bike racks at a park. Each bike rack has 4 bikes. How many bikes are there in all?
Answer:
3×4= 12 bikes.
Explanation:
As there are 3 bike racks and each rack has 4 bikes, so the number of bikes are there is 3×4= 12.
To represent this in the number line, we will start from 0 then skip the count by 4 three times. So the number of jumps is 3 and the size of the jump is 4.
Question 8.
DIG DEEPER!
You dig 8 holes. You plant 2 flower bulbs in each hole. You have5 bulbs left. How many flower bulbs did you have to start?
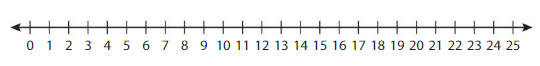
Answer:
3×5= 15 flower bulbs.
Explanation:
The number of holes dug is 8 and in each hole, 2 flower bulbs are planted, so the number of flower bulbs planted is 2×5= 10 and still, there are 5 bulbs left. So the total number of flower bulbs is 10+5= 15. To represent this in the number line, as there are 5 bulbs added then it will be 3 groups of 5 which is 3×5= 15. So we will start from 0 then skip the count by 5 three times and the number of jumps is 3 and the size of the jump is 5.
Use Number Lines to Multiply Homework & Practice 1.2
Question 1.
Find 3 × 6
Number of jumps: _____
Size of each jump: ______
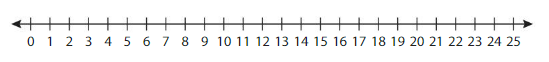
3 × 6 = _____
Answer:
3 × 6= 18
Number of jumps: 3
Size of each jump: 6.
Explanation:
To represent this in the number line, we will start from 0 then skip the count by 6 three times. So the number of jumps is 3 and the size of the jump is 6.
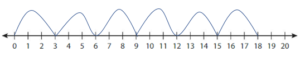
Question 2.
Find 4 × 5
Number of jumps: _____
Size of each jump: ______
4 × 5 = _____
Answer:
4 × 5= 20
Number of jumps: 4
Size of each jump: 5.
Explanation:
To represent this in the number line, we will start from 0 then skip the count by 5 four times. So the number of jumps is 4 and the size of the jump is 5.

Question 3.
Structure
Complete the multiplication equations in two different ways. Model each equation on the number line.
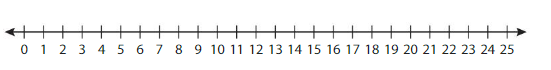
____ × ____ = 12
Answer:
2×6= 12
Explanation:
To represent this in the number line, we will start from 0 then skip the count by 6 two times. So the number of jumps is 2 and the size of the jump is 6.
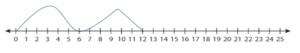
____ × ____ = 12
Answer:
4×3= 12
Explanation:
To represent this in the number line, we will start from 0 then skip the count by 3 four times. So the number of jumps is 4 and the size of the jump is 3.

Question 4.
Writing
Explain how you can use a number line to find 5 × 3.
Answer:
5×3= 15.
Explanation:
To represent this in the number line, we will start from 0 then skip the count by 3 five times. So the number of jumps is 5 and the size of the jump is 3.

Question 5.
Modeling Real Life
You have6 boxes of blueberry muffins. Each box has 4 muffins. How many muffins do you have in all?

Answer:
6×4= 24.
Explanation:
As we have 6 boxes of blueberry muffins with 4 muffins in each box, which means 6×4 = 24 muffins do we have it all.
Question 6.
DIG DEEPER!
You fill 8 pages of a photo album. Each page has 3 photos. You have one photo left. How many photos did you have to start?
Answer:
25 photos.
Explanation:
The total number of pages in a photo album is 8 and each page contains 3 photos, which means 8×3= 24, so there will be a total of 24 photos. Now 1 photo left, which means 24+1= 25. So there will be a total of 25 photos.
Review & Refresh
Question 7.
9 + 8 + 2 = _____
Answer:
19.
Explanation:
On adding 9+8+2 we will get the sum as 19.
Question 8.
6 + 5 + 3 = _____
Answer:
14.
Explanation:
On adding 6+5+3 we will get the sum as 14.
Question 9.
7 + 4 + 7 = ______
Lesson 1.3 Use Arrays to Multiply
Explore and Grow
Question 1.
Put 24 counters into equal rows. Draw your model.
Answer:
6×4= 24.
rows and 4 columns.
Explanation:
To keep 24 counters into equal rows, we will find the factors of 24 and then we can put 24 counters into equal rows. The factors of 24 are 2,3,4,6,8,12. Now we can pick any of the numbers from the factors of 24 and then put these 24 counters in equal groups. Let’s take six equal rows and arrange 24 counters in four equal rows as shown in the below image.

Question 2.
Put 24 counters into a different number of equal rows. Draw your model.
Answer:
Question 3.
Structure Compare your models. How are the models the same? How are they different?
Answer:
Think and Grow: Multiplication and Arrays
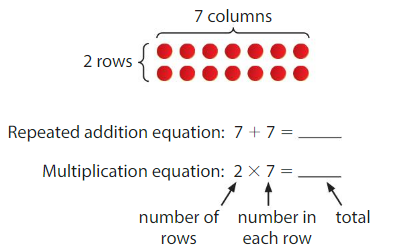
An array is a group of objects organized into rows and columns. Each row has the same number of objects.
Example
How many counters are there in all?
Show and Grow
Question 1.
How many counters are there in all?
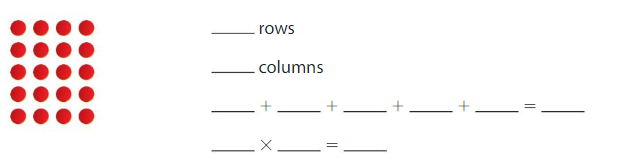
Answer:
5 rows and 4 columns,
5+5+5+5= 20,
5×4= 20.
The total number of counters is 20.
Explanation:
From the above image, we can see there are 5 rows and 4 columns. The product is 5×4= 20 and the sum is 5+5+5+5= 20.
Question 2.
Draw an array to multiply 6 × 3.
6 × 3 = ______
Answer:
6 × 3 = 18
Explanation:
To draw an array of 6 × 3, we will place 6 rows and 3 columns. By that, we can form an array of 6×3 which is 18.

Apply and Grow: Practice
Draw an array to multiply.
Question 3.
4 × 8 = _____
Answer:
4 × 8 = 32
Explanation:
To draw an array of 4 × 8, we will place 4 rows and 8 columns. By that, we can form an array of 4×8 which is 32.

Question 4.
3 × 9 = ____
Answer:
3×9= 27
Explanation:
To draw an array of 3×9, we will place 3 rows and 9 columns. By that, we can form an array of 3×9 which is 27.

Question 5.
7 × 3 = _____
Answer:
7 × 3 = 21
Explanation:
To draw an array of 7×3, we will place 7 rows and 3 columns. By that, we can form an array of 7×3 which is 21.

Question 6.
6 × 5 = _____
Answer:
6×5= 30
Explanation:
To draw an array of 6×5, we will place 6 rows and 5 columns. By that, we can form an array of 6×5 which is 30.

Question 7.
Number Sense
Newton has a 2 × 10 array of baseballs. He adds another row. How many baseballs does he add? Write a multiplication equation for his new array.

He adds ______ baseballs
____ × _____ = _____
Answer:
Newton adds 30 baseballs.
3×10= 30.
Explanation:
As Newton has an array of 2 × 10 of baseballs which are 20 baseballs as he adds another row, which means 3×10 of baseballs which are 30 baseballs. So he adds 30 and 20 which is 10 baseballs Newton was added and the multiplication equation for his new array is 3×10 which is 30 baseballs.
Question 8.
DIG DEEPER!
Use 6 counters to make as many different arrays as possible using all of the counters. Draw the arrays. Then write a multiplication equation for each array.

Answer:
2×3= 6,
3×2= 6.
Explanation:
The different possible arrays for 6 counters are 2×3= 6 which has 2 rows and 3 columns and 3×2= 6 which has 3 rows and 2 columns.
 This array consists of 2 rows and 3 columns
This array consists of 2 rows and 3 columns
 This array consists of 3 rows and 2 columns.
This array consists of 3 rows and 2 columns.
Think and Grow: Modeling Real Life
A phone has 6 rows of apps with 4 apps in each row. How many apps are on the phone?
Draw:
Equation:
There are _____ apps on the phone.
Answer:
6×4= 24
Explanation:
As a phone has 6 rows of apps with 4 apps in each row, The number of apps on the phone are 6×4= 24.
Show and Grow
Question 9.
Your classroom has 3 rows of desks with 10 desks in each row. How many desks are in your classroom?
Answer:
3×10= 30 desks.
Explanation:
The number of rows in the classroom is 3 rows with 10 desks in each row, which means 3×10= 30 desks in the classroom.
Question 10.
DIG DEEPER!
A square array has an equal number of rows and columns. A farmer has 9 corn seeds to plant in a square array. Draw the square array the farmer can use to plant all of the seeds. How many rows and columns are there?

Answer:
3×3= 9.
Explanation:
As the farmer has 9 corn seed to plant in a square array, so the possible square array is with 3 rows and 3 columns, which is 3×3= 9.

Use Arrays to Multiply Homework & Practice 1.3
Question 1.
How many counters are there in all?
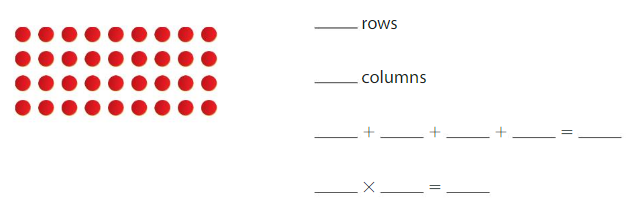
Answer:
4×9= 36 counters.
4 rows, 9 columns.
9+9+9+9= 36.
Explanation:
In the above image, we can see 4 rows and 9 columns which makes 36 counters. The product is 4×9= 36 and the sum is 9+9+9+9= 36.
Draw an array to multiply.
Question 2.
9 × 2 = _____
Answer:
9×2= 18.
Explanation:
This array contains 9 rows and 2 columns which makes 18 counters. The product is 9×2= 18 and the sum is
9+9= 18.

Question 3.
4 × 5 = _____
Answer:
4×5= 20.
Explanation:
This array contains 4 rows and 5 columns which makes 20 counters. The product is 4×5= 20 and the sum is
5+5+5+5= 20.

Question 4.
YOU BE THE TEACHER
Descartes has 24 counters. He says he can use all the counters to make an array with 3 rows. Is he correct? Explain.

Answer:
With 3 rows and 8 columns, 24 counters can be made.
Explanation:
Yes, he is correct. With 3 rows and 8 columns, he can make 24 counters. The product of the array is 3×8= 24 and the sum of the array is 8+8+8= 24.
Question 5.
Number Sense
Newton has a 4 × 8 array of dominoes. He adds 2 more rows. How many dominoes does he add? Write a multiplication equation for his new array.
He adds _____ dominoes
_____ × ____ = _____
Answer:
Newton adds 16 dominoes,
6×8= 48.
Explanation:
As Newton has a 4×8 array of dominoes which is 32 dominoes and he adds 2 more rows, which is 4+2= 6. Then the dominoes will be a 6×8 array. And the multiplication equation for Newton’s new array is 6×8= 48. So the number of dominoes added is 48-32 which is 16 dominoes he was added.
Question 6.
Modeling Real Life
An art teacher hangs 2 rows of paintings with 10 paintings in each row. How many paintings does she hang?
Answer:
20 paintings.
Explanation:
As an art teacher hangs 2 rows of paintings in each row, so the number of paintings is 2×10= 20 paintings.
Question 7.
DIG DEEPER!
A museum has 16 shark teeth to display in a square array. Draw the square array the museum can use to display all of the teeth. How many rows and columns are there?

Answer:
There are 4 rows and 4 columns.
Explanation:
As the museum has 16 shark teeth to display in a square array, so the array can be written as 4×4= 16 which has 4 rows and 4 columns.

Review & Refresh
Complete the equation
Question 8.
3 + 8 = 8 + ____
Answer:
3+8= 8+3
Explanation:
By the commutative property of addition, we can change the order of the addends which does not change the sum. So 3+8= 8+3.
Question 9.
10 + 0 = ____ + 10
Answer:
10+0= 0+10.
Explanation:
By the commutative property of addition, we can change the order of the addends which does not change the sum. So 10+0= 0+10.
Question 10.
6 + ____ = 7 + 6
Answer:
6+7= 7+6.
Explanation:
By the commutative property of addition, we can change the order of the addends which does not change the sum. So 6+7= 7+6.
Question 11.
____ + 8 = 8 + 9
Answer:
9+8= 8+9.
Explanation:
By the commutative property of addition, we can change the order of the addends which does not change the sum. So 9+8= 8+9.
Lesson 1.4 Multiply in Any Order
Explore and Grow
Question 1.
Write the multiplication equation for the array. Turn your paper and write the equation for the array.
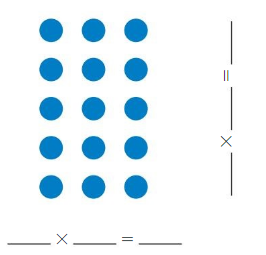
Answer:
5×3= 15.
Explanation:
In the above image, we can see 5 rows and 3 columns. So the product of the array is 5×3= 15.
Structure
Compare the equations. How are they the same? How are they different?
Think and Grow: Commutative Property of Multiplication
Ina multiplication equation, the numbers that are multiplied are called factors. The answer is called the product.
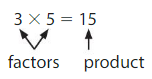
Commutative Property of Multiplication: Changing the order of factors does not change the product.
Example
Complete the statements
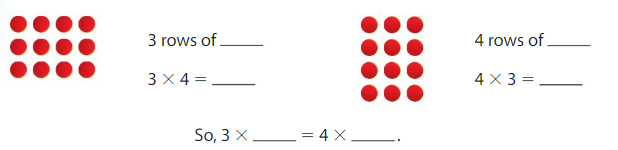
Show and Grow
Question 1.
Complete the statements
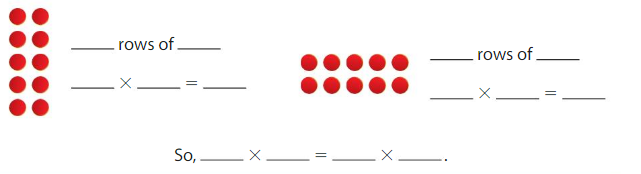
Answer:
5×2= 2×5.
Explanation:
In the first image, we can see 5 rows and 2 columns, this means 5 rows of 2. And in the second image, we can 2 rows and 5 columns, this means 2 rows of 5. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 5×2= 2×5.
Question 2.
Draw an array to show the Commutative Property of Multiplication. Complete the statements.

____ × ____ = _____
_____ × ____ = _____
So, ____ × _____ = ____ × ____
Answer:
2×3= 6,
3×2= 6.
So, 2×3= 3×2.
Explanation:
In the above image, we can see 2 rows and 3 columns, this means 2 rows of 3. And we can also write as 3 rows and 2 columns as we can see in the given below image. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 2×3= 3×2.

Apply and Grow: Practice
Draw an array to show the Commutative Property of Multiplication. Complete the statements.
Question 3.
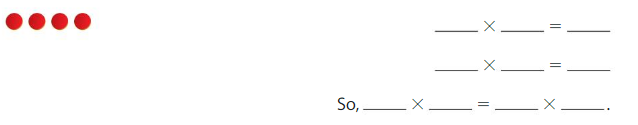
Answer:
1×4= 4,
4×1= 4.
So, 1×4= 4×1.
Explanation:
In the above image, we can see 1 row and 4 columns. Which makes 1 row of 4. And we can also write as 4 rows and 1 column as we can see in the given below image. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 1×4= 4×1.
![]()
Question 4.
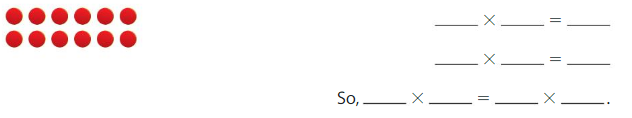
Answer:
2×6= 12,
6×2= 12,
2×6= 6×2.
Explanation:
In the above image, we can see 2 rows and 6 columns. Which makes 2 rows of 6. And we can also write as 6 rows and 2 columns as we can see in the given below image. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 2×6= 6×2.

Question 5.
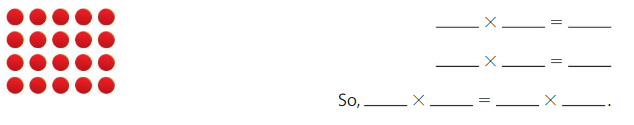
Answer:
4×5= 20,
5×4= 20.
So, 4×5= 5×4.
Explanation:
In the above image, we can see 4 rows and 5 columns. Which makes 4 rows of 5. And we can also write as 5 rows and 4 columns as we can see in the given below image. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 4×5= 5×4.

Complete the equation.
Question 6.
8 × 3 = 3 × ____
Answer:
8×3= 24,
3×8= 24.
So, 8×3= 3×8.
Explanation:
By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, we can also write as 3 rows and 8 columns as we can see in the given below image which is 8×3= 3×8.

Question 7.
10 × 2 = ____ × 10
Answer:
10×2 = 20,
2×10= 20.
So, 10×2= 2×10.
Explanation:
By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, we can also write as 2 rows and 10 columns as we can see in the given below image which is 10×2= 2×10.

Question 8.
1 × ____ = 9 × 1
Answer:
1×9= 9,
9×1= 9.
So, 1×9= 9×1.
Explanation:
By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, we can also write as 9 rows and 1 column which is 1×9= 9×1.
Question 9.
Structure
Which shape completes the equation?

Answer:
Moon shape.
Explanation:
By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, the missing shape is the moon.
Think and Grow: Modeling Real Life
Your friend makes 7 rows of 6 stickers. You want to put the same number of stickers into 6 rows. How many stickers do you put in each row? Explain.
You put ____ stickers in each row.
Explain.
Answer:
7×6= 42,
6×7= 42.
So, 7×6= 6×7.
You can put 7 stickers in each row.
Explanation:
The number of stickers made is 7 rows of 6 stickers and want to put the same number of stickers into 6 rows. So, by the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, we can make 6 rows of 7 stickers, which has 6 rows and 7 columns.
Show and Grow
Question 10.
Your friend makes 9 rows of 4 award ribbons. You want to put the same number of award ribbons into 4 rows. How many award ribbons do you put in each row? Explain

Answer:
9×4= 36,
4×9= 36.
So, 9×4= 4×9.
So 9 award ribbons can be put in each row.
Explanation:
Given, 9 rows of 4 award ribbons and we need to put the same number of award ribbons into 4 rows. So by the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So, we can make 4 rows of 9 award ribbons, which has 4 rows and 9 columns and 9 award ribbons can be put in each row.
Question 11.
DIG DEEPER!
You have 2 rows of 8 toy cars. Your friend has 5 rows of 2 toy cars. How can you use the Commutative Property of Multiplication to find how many rows your friend needs to add so that you both have the same number of toy cars?

Answer:
Explanation:
Multiply in Any Order Homework & Practice 1.4
Question 1.
Complete the statements.
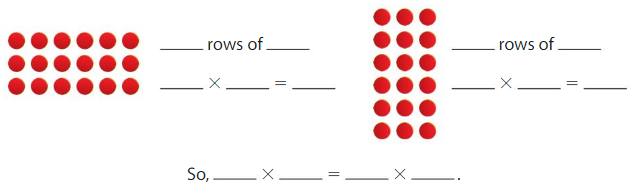
Answer:
3 rows of 6,
3×6= 18.
6 rows of 3
6×3= 18,
So, 3×6= 6×3.
Explanation:
In the first image, we can see 3 rows and 6 columns, this means 3 rows of 6. And in the second image, we can 6 rows and 3 columns, this means 6 rows of 3. By the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. So 3×6= 6×3.
Question 2.
Draw an array to show the Commutative Property of Multiplication. Complete the statements.
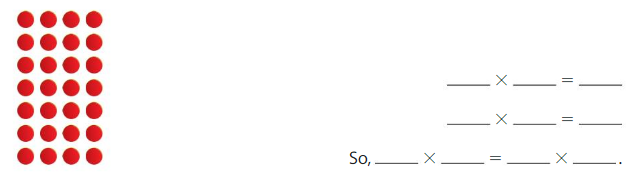
Answer:
7×4= 28,
4×7= 28.
So, 7×4= 4×7
Explanation:
In the image, we can see 7 rows and 4 columns, this means 7 rows of 4. And by the commutative property of multiplication, we can state that the order in which we multiply the numbers does not change the product. To draw an array by the Commutative Property of Multiplication, we will take 4 rows of 7, which means 4 rows and 7 columns as in the below image. So, 7×4= 4×7.

Which two arrays can you use to show the Commutative Property of Multiplication?
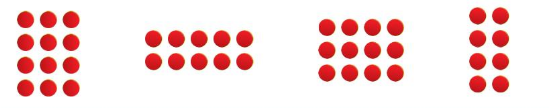
Answer:
The first image and third image shows the commutative property of multiplication.
Explanation:
From the above image, the first and third arrays show the Commutative property of multiplication. Which states that the order in which we multiply the numbers does not change the product. As in the first image, we can see 4 rows of 3 which have 4 rows and 3 columns and in the third image, we can see 3 rows of 4 which has 3 rows and 4 columns.
Question 4.
Precision
Write two equations that show the Commutative Property of Multiplication.
____ × ____ = ____ × _____
____ × _____ = ____ × ____
Answer:
5×6= 6×5,
2×3= 3×2.
Explanation:
The Commutative property of multiplication states that the order in which we multiply the numbers does not change the product. For example, if we take 5×6= 30 then the commutative property is 6×5= 30.
So 5×6= 6×5.
Question 5.
Modeling Real Life
A computer lab has 6 rows of 5 computers. A technology teacher wants to rearrange the computers into5 rows. How many computers does the teacher put in each row? Explain.
Answer:
6 computers do the teacher put in each row.
Explanation:
As a computer lab has 6 rows of 5 computers, and the technology teacher rearranged the computers into 5 rows, so the teacher will put 6 computers by commutative property as it states that the order in which we multiply the numbers does not change the product. So 6×5= 5×6.
Question 6.
DIG DEEPER!
You have 6 rows of 4 pennies. Your friend has 2 rows of 6 pennies. How many rows does your friend need to add so that you both have the same number of pennies?

Answer:
So, a friend needs 2 rows to add then both will have the same number of pennies.
Explanation:
There are 6 rows of 4 pennies, which is 6 rows and 4 columns and the friend has 2 rows of 6 pennies which is 2 rows and 6 columns. So my friend needs to add 2 rows and then it will be 2 rows+2 rows= 4 rows and there will be 6 columns. So, by the commutative property, as it states that the order in which we multiply the numbers does not change the product. So, 6×4= 4×6.
Review & Refresh
Question 7.
Newton hits a ball 5 fewer times than Descartes does. Newton hits the ball 9 times. How many times does Descartes hit the ball?
Answer:
Explanation:
Lesson 1.5 Divide: Size of Equal Groups
Explore and Grow
Question 1.
Put 18 counters in 6 equal groups. Draw to show your groups
Number of counters in each group: _____
Answer:
The number of counters in each group is 3 counters.
Explanation:
To put 18 counters in 6 equal groups, we will divide 18 by 6 then the result is 3. So, we can put counters in 3 counters in 6 equal groups as shown in the given below image.

Question 2.
Put 18 counters in 3 equal groups. Draw to show your groups
Number of counters in each group: _____
Answer:
The number of counters in each group is 6.
Explanation:
To put 18 counters in 3 equal groups, we will divide 18 by 3 then the result is 6. So, we can put counters in 6 counters in 3 equal groups as shown in the given below image.

Structure
How does changing the number of equal groups change the number of counters in each group?
Answer:
Explanation:
Think and Grow: Using Equal Groups to Divide
Division is an operation that gives the size of equal groups or the number of equal groups. When you know the total number of objects and the number of equal groups, you can divide to find the size of each group.3 equal groups
Example
Divide 12 counters into. How many counters are in each group?
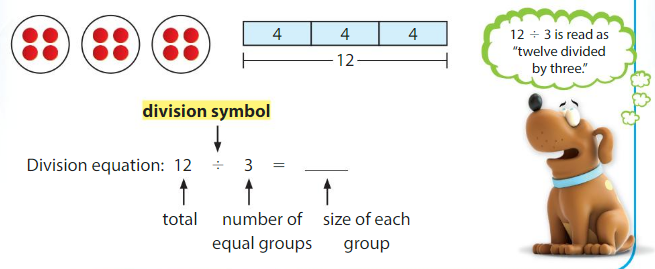
Show and Grow
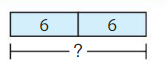
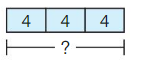
Question 1.
Divide 15 counters into5 equal groups. How many counters are in each group?
15 ÷ 5 = ____
Answer:
The number of counters placed in 5 equal groups is 3 counters.
Explanation:
There are 15 counters and we need to place those 15 counters in 5 equal groups. So we will divide 15 by 5, 15÷5= 3. So we will pace 3 counters in 5 equal groups.

Use the tape diagram to model the equation.
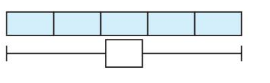
Answer:
The number of counters placed in 5 equal groups is 3 counters.
Explanation:
There are 15 counters and we need to place those 15 counters in 5 equal groups. So we will divide 15 by 5, 15÷5= 3. So we will pace 3 counters in 5 equal groups.
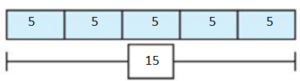
Apply and Grow: Practice
Question 2.
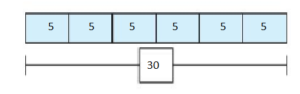
Divide 30 counters into 6 equal groups. How many counters are in each group?
30 ÷ 6 = ____
Answer:
The number of counters placed in 6 equal groups is 5 counters. AS 30÷6= 5.
Explanation:
There are 30 counters and we need to place those 30 counters in 6 equal groups. So we will divide 30 by 6,
30÷6= 5. So we will pace 5 counters in 6 equal groups.

Use the tape diagram to model the equation.
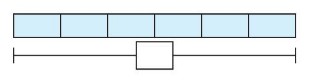
Answer:
The number of counters placed in 6 equal groups is 5 counters. AS 30÷6= 5.
Explanation:
There are 30 counters and we need to place those 30 counters in 6 equal groups. So we will divide 30 by 6,
30÷6= 5. So we will pace 5 counters in 6 equal groups.
Question 3.
Divide 16 counters into 2 equal groups. How many counters are in each group?
16 ÷ 2 = ____
Answer:
16÷2= 8.
Explanation:
There are 16 counters and we need to place those 16 counters in 2 equal groups. So we will divide 16 by 2,
16÷2= 8. So we will pace 8 counters in 2 equal groups.
Question 4.
Divide 9 counters into 3 equal groups. How many counters are in each group?
9 ÷ 3 = ____
Answer:
9 ÷ 3= 3.
Explanation:
There are 9 counters and we need to place those 9 counters in 3 equal groups. So we will divide 9 by 3,
9÷3= 3. So we will pace 3 counters in 3 equal groups.
Question 5.
Structure
Write the division equation that matches the tape diagram.
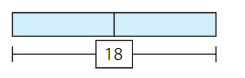
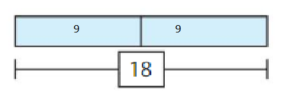
____ ÷ ____ = ____
Answer:
18÷2= 9.
Explanation:
There are 18 counters and we need to place those 18 counters in 2 equal groups. So we will divide 18 by 2,
18÷2= 9. So we will pace 9 counters in 2 equal groups.
Question 6.
DIG DEEPER!
Newton has a tennis ball collection. He can divide the balls into3 equal groups with none left over. He can also divide the balls into4 equal groups with none left over. How many tennis balls does he have?
Answer:
Think and Grow: Modeling Real Life
You have 30 seashells. You put an equal number of seashells in 5 bags. How many seashells are in each bag?

Model:
Division equation:
There are _____ seashells in each bag.
Answer:
Division equation: 30÷5= 6.
There are 6 seashells in each bag.
Explanation:
As there are 30 seashells and we need to put an equal number of seashells in 5 bags, we will divide 30÷5= 6. So we will 6 seashells in each bag.
Show and Grow
Question 7.
You have 28 rocks. You put an equal number of rocks in 4 piles. How many rocks are in each pile?
Answer:
28÷4= 7.
Explanation:
There are 28 rocks and we should put an equal number of rocks in 4 piles, we will divide 28 by 4. So 28÷4= 7. So there will be 7 rocks in each pile.
Question 8.
DIG DEEPER!
Newton and Descartes each have40 quarters. Newton puts his quarters into5 equal groups. Descartes puts his quarters into4 equal groups. Who has more quarters in each group?

Answer:
Descartes has more quarters than Newton.
Explanation:
As both Newton and Descartes have 40 quarters and Newton puts his quarters into 5 equal groups, which means 40÷5= 8. Newton put his 8 quarters into 5 equal groups and Descartes puts his quarters into 4 equal groups, which means 40÷4= 10. Descartes put his 10 quarters into 4 equal groups. So, Descartes has more quarters than Newton.
Divide: Size of Equal Groups Homework & Practice 1.5
Question 1.
Divide 16 counters into 4 equal groups. How many counters are in each group?
16 ÷ 4 = ____
Answer:
16÷4= 4.
Explanation:
There are 16 counters and we need to place those 16 counters in 4 equal groups. So we will divide 16 by 4,
16÷4= 4. So we will pace 4 counters in 4 equal groups.



Use the tape diagram to model the equation.
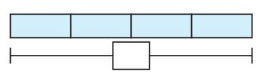
Answer:
16÷4= 4.
Explanation:
There are 16 counters and we need to place those 16 counters in 4 equal groups. So we will divide 16 by 4,
16÷4= 4. So we will pace 4 counters in 4 equal groups.
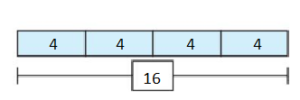
Question 2.
Divide 28 counters into 7 equal groups. How many counters are in each group?
28 ÷ 7 = ____
Answer:
28 ÷ 7= 4.
Explanation:
There are 28 counters and we need to place those 28 counters in 7 equal groups. So we will divide 28 by 7,
28 ÷ 7= 4. So we will pace 4 counters in 7 equal groups.
Question 3.
Divide 27 counters into 3 equal groups. How many counters are in each group?
27 ÷ 3 = ____
Answer:
27 ÷ 3 = 9.
Explanation:
There are 27 counters and we need to place those 27 counters in 3 equal groups. So we will divide 27 by 3,
27 ÷ 3= 9. So we will pace 9 counters in 3 equal groups.
Question 4.
YOU BE THE TEACHER
Newton says you divide 18 counters into 3 equal groups. Is he correct?

Answer:
Yes, Newton is correct.
Explanation:
Yes, Newton is correct. As we can see in the above image he divided 18 counters into 3 equal groups with different numbers of counters in each group.
Question 5.
Precision
A class has 14 boys and 18 girls. Can the teacher divide the class equally into 4 groups with no students remaining? Explain.
Answer:
The teacher places 8 students in 4 equal groups with no students remaining.
Explanation:
As a class has 14 boys and 18 girls, so the total number of students is 14+18= 32 students. As the teacher divided the class equally into 4 groups, 32÷4= 8. So the teacher places 8 students in each group with no students remaining.
Question 6.
Modeling Real Life
You have 14 erasers. You and your friend share them equally. How many erasers do you and your friend each get?

Answer:
7 erasers will get each.
Explanation:
There are 14 erasers and those erasers are shared equally among two of them, so we will divide 14 by 2 which is 14÷2= 7. So 7 erasers will get each.
Question 7.
DIG DEEPER!
Newton and Descartes each have 42 glow-in-the-dark stickers. Newton divides into 6 equal groups. Descartes divides his into7 equal groups. Who has more stickers in each group?
Answer:
Newton has more stickers than Descartes in each group.
Explanation:
As Newton and Descartes, each have 42 glow-in-the-dark stickers and Newton divides them into 6 equal groups, which is 42÷6= 7. So Newton has 7 glow-in-the-dark stickers in 6 equal groups. And Descartes divides his into7 equal groups which is 42÷7= 6. So Descartes has 6 glow-in-the-dark stickers in 7 equal groups. Newton has more stickers than Descartes in each group.
Review & Refresh
Question 8.
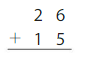
Answer:
41
Explanation:
On adding 26+15 we will get 41.
Question 9.
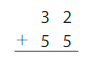
Answer:
87.
Explanation:
On adding 32+55 we will get 87.
Question 10.
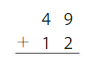
Answer:
61.
Explanation:
On adding 49+12 we will get 61.
Question 11.
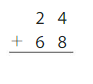
Answer:
92.
Explanation:
On adding 24+68 we will get 92.
Lesson 1.6 Divide: Number of Equal Groups
Explore and Grow
Question 1.
Put 24 counters in equal groups of 4. Draw to show your groups.
Number of groups: ______
Answer:
The number of groups is 6.
Explanation:
To put 24 counters in equal groups of 4, we will divide 24 by 4, 24÷4= 6. So we will put 4 counters in 6 equal number of group.






Question 2.
Put 24 counters in equal groups of 6. Draw to show your groups.
Number of groups: ______
Answer:
The number of groups is 4.
Explanation:
To put 24 counters in equal groups of 6, we will divide 24 by 6, 24÷6= 4. So we will put 6 counters in 4 equal number of group.

Structure
How does changing the size of the groups change the number of equal groups?
Think and Grow: Using Equal Groups to Divide
When you know the total number of objects and the size of each group, you can divide to find the number of equal groups.
Example
Divide 12 counters into. How many groups are there?
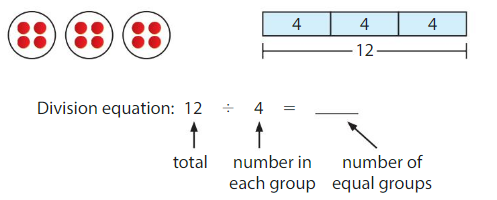
Show and Grow
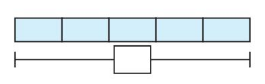
Question 1.
Divide 10 counters into groups of 2. How many groups are there?
10 ÷ 2 = ____
Answer:
10 ÷ 2 = 5.
Explanation:
To put 10 counters in equal groups of 2, we will divide 10 by 2, 10÷2= 5. So we will put 2 counters in 5 equal number of group.
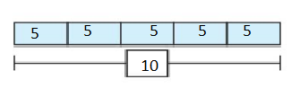
Use the tape diagram to model the equation.
Answer:
10÷2= 5.
Explanation:
To put 10 counters in equal groups of 2, we will divide 10 by 2, 10÷2= 5. So we will put 2 counters in 5 equal number of group.
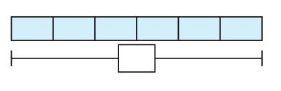
Question 2.
Divide 24 counters into groups of 4. How many groups are there?

24 ÷ 4 = ____
Answer:
24÷4= 6.
Explanation:
To put 24 counters in equal groups of 4, we will divide 24 by 4, 24÷4= 6. So we will put 4 counters in 6 equal number of group.
Use the tape diagram to model the equation.
Answer:
24÷4= 6.
Explanation:
To put 24 counters in equal groups of 4, we will divide 24 by 4, 24÷4= 6. So we will put 4 counters in 6 equal number of group.
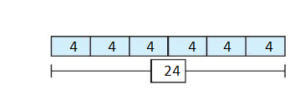
Apply and Grow: Practice
Question 3.
Divide 30 counters into groups of 6. How many groups are there?

30÷6=
Use the tape diagram to model the equation.
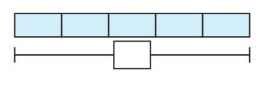
Answer:
30÷6= 5.
Explanation:
To put 30 counters in equal groups of 6, we will divide 30 by 6, 30÷6= 5. So we will put 5 counters in 6 equal number of group.
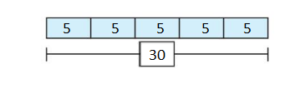
Question 4.
Divide 15 counters into groups of 5. How many groups are there?
15 ÷ 5 = _____
Answer:
15÷5= 3.
Explanation:
To put 15 counters in equal groups of 5, we will divide 15 by 5, 15÷5= 3. So we will put 3 counters in 5 equal number of group.
Question 5.
Divide 16 counters into groups of 4. How many groups are there?
16 ÷ 4 = _____
Answer:
16÷4= 4.
Explanation:
To put 16 counters in equal groups of 4, we will divide 16 by 4, 16÷4= 4. So we will put 4 counters in 4 equal number of group.
Question 6.
Structure
You want to bake as many loaves of banana bread as possible with 12 eggs. Each loaf of bread requires 2 eggs. Which models can you use to find how many loaves of bread you can make?
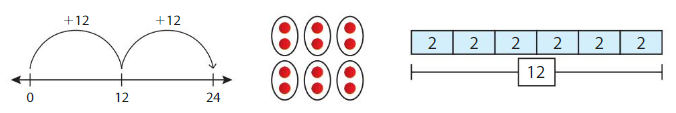
Answer:
Explanation:
Think and Grow: Modeling Real life
A florist uses 35 roses to make bouquets. Each bouquet has 7 roses. How many bouquets does the florist make?
Equation:
Model:

The florist makes ______ bouquets
Answer:
Equation: 35÷7= 5
Explanation:
As the florist has 35 roses to make bouquets and each bouquet has35 7 roses, so the florist can make 35÷7= 5 bouquets.
Show and Grow
Question 7.
A farmer puts 48 eggs into cartons. He puts 6 eggs in each carton. How many cartons does he use?
Answer:
8 cartons.
Explanation:
As the farmer puts 48 eggs into cartons and in each carton, there are 6 eggs. So the farmer uses 48÷6= 8 cartons.
Question 8.
DIG DEEPER!
Newton uses his subway pass 3 times each day. Descartes uses his pass 2 times each day. Who will use all of his rides first? Explain.
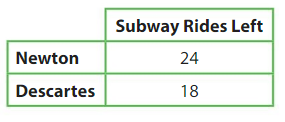
Would your answer change if Newton and Descartes both use their passes 3 times each day? Explain.
Answer:
Newton will finish his rides first then Descartes.
Descartes will use all of his rides first if Descartes use passes 3 times each day.
Explanation:
As Newton has 24 subway rides left and he uses subway pass 3 times each day, so the number of rides left are
24-3= 21. Descartes has 18 subway rides and he uses his pass 2 times each day, so the number of rides left are
18-2= 16. By using repeated subtraction which starts from 24 as given and then we will subtract 3 until we get 0.
24-3= 21
21-3= 18
18-3= 15
15-3= 12
12-3= 9
9-3= 6
6-3= 3
3-3=0.
So, Newton will complete the subway pass by 8 times.
18-2= 16
16-2= 14
14-2= 12
12-2= 10
10-2= 8
8-2= 6
6-2= 4
4-2= 2
2-2= 0.
And Descartes will complete the subway pass by 9 times. So Newton will use all his rides first.
And if Descartes uses 3 times of his pass then
18-3= 15
15-3= 12
12-3= 9
9-3= 6
6-3= 3
3-3= 0.
Descartes will complete the subway pass by 6 times, So Descartes will complete his pass first.
Divide: Number of Equal Groups Homework & Practice 1.6
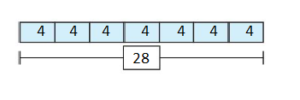
Question 1.
Divide 28 counters into groups of 4. How many groups are there?

28 ÷ 4 = ____
Answer:
28 ÷ 4 =7
Explanation:
To put 28 counters in equal groups of 4, we will divide 28 by 4, 28÷4= 7. So we will put 4 counters in 7 equal number of group.
Use the tape diagram to model the equation.
Answer:
28÷4= 7.
Explanation:
To put 28 counters in equal groups of 4, we will divide 28 by 4, 28÷4= 7. So we will put 4 counters in 7 equal number of group.
Question 2.
Divide 25 counters into groups of 5. How many groups are there?
25 ÷ 5 = _____
Answer:
25 ÷ 5 = 5.
Explanation:
To put 25 counters in equal groups of 5, we will divide 25 by 5, 25÷5= 5. So we will put 5 counters in 5 equal number of group.
Question 3.
Divide 12 counters into groups of 6. How many groups are there?
12 ÷ 6 = _____
Answer:
12 ÷ 6 = 2.
Explanation:
To put 12 counters in equal groups of 6, we will divide 12 by 6, 12÷6= 2. So we will put 2 counters in 6 equal number of group.
Question 4.
Writing
Write and solve a problem in which you need to find the number of equal groups.
Answer:
Divide 22 counters into groups of 11. How many groups are there?
Explanation:
To put 22 counters in equal groups of 11, we will divide 22 by 11, 22÷11= 2. So we will put 2 counters in 11 equal number of group.
Question 5.
Reasoning
Your classroom has 30 chairs that need to be stacked with 5 chairs in each stack. Your teacher already made 2 stacks. How many stacks of chairs still need to be made?
Answer:
The number of stacks of chairs that still need to be made is 4.
Explanation:
As a classroom has 30 chairs and that needed to be stacked with 5 chairs in each stack, so 30÷5= 6 stacks. And the teacher already made 2 stacks, which means 6-2= 4. So the number of stacks of chairs that still need to be made are 4 stacks.
Question 6.
DIG DEEPER!
You have more than 30 and fewer than 40 piñata toys. You divide them into groups with 8 in each group. How many groups do you make?

Answer:
There will be 4 groups with 8 pinata toys in each group.
Explanation: As there are more than 30 and fewer than 40 pinata toys, and we need to divide them into groups with 8 in each group. So we need to choose the number between 30-40. As we can see fewer than 40 and more than 30, so we will choose 32 as it will divide them into groups with 8 in each group, 32÷8= 4. So there will be 4 groups with 8 pinata toys in each group.
Question 7.
A street vendor puts 42 apples into baskets. She puts 6 apples in each basket. How many baskets does she use?
Answer:
7 baskets.
Explanation:
As a street vendor puts 42 apples into baskets and she puts 6 apples in each basket, so she needs 42÷6= 7 baskets.
Question 8.
DIG DEEPER!
Newton and Descartes are at an amusement park. Newton uses 2 tickets to ride each roller coaster. Descartes uses 3 tickets to ride at the front of each roller coaster. Who runs out of tickets first? Explain.
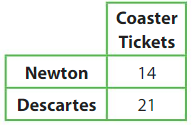
Newton Descartes, They run out of tickets at the same time
Answer:
Newton will run out of tickets first, as he has fewer tickets.
Explanation:
As Newton has 14 coaster tickets and uses 2 tickets to ride each roller coaster, so there will 14-2= 12 tickets remaining. And Descartes has 21 coaster tickets and uses 3 tickets to ride, so the remaining tickets are 21-3= 18 tickets. Newton will run out of tickets first, as he has fewer tickets.
Review & Refresh
Find the difference. Use addition to check your answer.
Question 9.
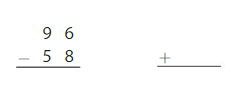
Answer:
96-58= 38,
38+58= 96.
Explanation:
On subtracting 96-58 we will get 38 and by adding 58 and 38 we will get 96. So by adding we can check your answer.
Question 10.
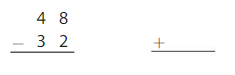
Answer:
48-32= 16,
16+32= 48.
Explanation:
On subtracting 48-32 we will get 16 and by adding 32 and 16 we will get 48. So by adding we can check your answer.
Lesson 1.7 Use Number Lines to Divide
Explore and Grow
Question 1.
Find the difference. Use each difference as the starting number in the next equation. Model the problems on the number line.
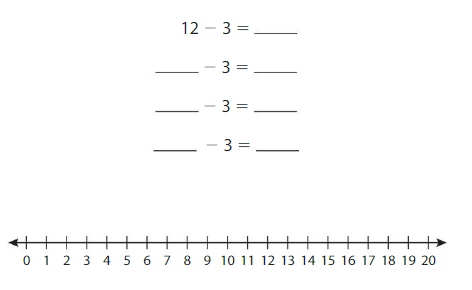
Answer:
12-3= 9,
9-3= 6,
6-3= 3,
3-3= 0.
Explanation:
To represent a number line, we will use repeated subtraction which starts from 12 as given and then we will subtract 3 until we get 0. So the number of jumps is 4 and the size of the jump is 3.
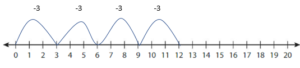
Question 2.
Structure
How can you use a number line to help you find 12 ÷ 3?
Answer:
12÷3= 4.
Explanation:
To use the number line for the given value 12÷3 which is 4, we will start from 0 then skip the count by 4 three times. So the number of jumps is 4 and the size of the jump is 3. And we can also use the subtraction method, which starts with 12 and subtract 3 until we get 0.
Think and Grow: Number Lines and Repeated Subtraction
Example Find 20 ÷ 4.
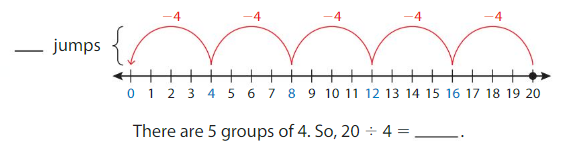
Another Way:
Use repeated subtraction. Start with 20. Subtract 4 until you reach 0.
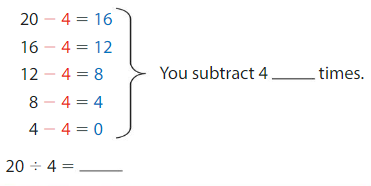
Show and Grow
Complete the equations.
Question 1.
24 ÷ 6 = ______
Answer:
24 ÷ 6 = 4.
Explanation:
By using the number line, we will countback by 6s from 24 until we reach 0,
24÷6= 4, so there are 4 groups of 6.
Question 2.
16 ÷ 8 = _____
16 – 8 = _____
____ – 8 = 0
Answer:
16 ÷ 8 = 3,
16-8= 8,
8-8= 0.
Explanation:
We will use repeated subtraction which starts from 16 as given and then we will subtract 8 until we get 0. So the number of jumps is 2 and the size of the jump is 8.
16 ÷ 8 = 3,
16-8= 8,
8-8= 0.
Question 3.
27 ÷ 9 = _____
27 – 9 = _____
____ – 9 = ____
_____ – 9 = 0
Answer:
27 ÷ 9 = 3,
27-9= 18,
18-9= 9,
9-9= 0.
Explanation:
We will use repeated subtraction which starts from 27 as given and then we will subtract 9 until we get 0. So the number of jumps is 3 and the size of the jump is 9.
27 ÷ 9 = 3,
27-9= 18,
18-9= 9,
9-9= 0.
Apply and Grow: Practice
Complete the equations.
Question 4.
25 ÷ 5 = _____
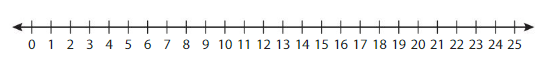
Answer:
25 ÷ 5 = 5.
Explanation:
By using the number line, we will countback by 5s from 25 until we reach 0,
25÷5= 5, so there are 5 groups of 5.
Question 5.
21 – 7 = _____
____ – 7 = _____
_____ – 7 = 0
____ ÷ _____ = ____
Answer:
21-7= 14,
14-7= 7,
7-7= 0,
21÷7= 3.
Explanation:
we will use repeated subtraction which starts from 21 as given and then we will subtract 7 until we get 0.
21-7= 14,
14-7= 7,
7-7= 0,
21÷7= 3.
Question 6.
36 – 9 = _____
____ – 9 = ____
____ – 9 = ____
_____ – 9 = 0
____ ÷ ____ = ____
Answer:
36-9= 27,
27-9= 18,
18-9= 9,
9-9= 0.
36÷9= 4.
Explanation:
we will use repeated subtraction which starts from 36 as given and then we will subtract 9 until we get 0.
36-9= 27,
27-9= 18,
18-9= 9,
9-9= 0.
36÷9= 4.
Question 7.
YOU BE THE TEACHER
Descartes uses a number line to find 18 ÷ 2. Is he correct? Explain.
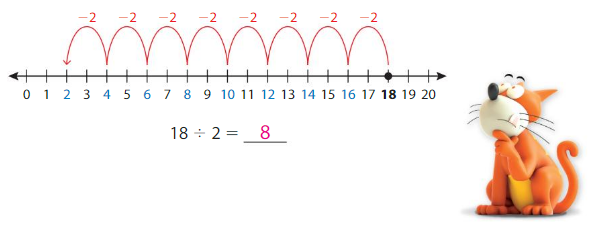
Explanation:
No, Descartes is not correct. As 18÷2= 9 and here the given result 8. So Descartes is incorrect.
Think and Grow: Modeling Real life
Each age group is divided into teams with 6 players on each team. Each team receives a trophy at the end of the season. How many trophies are needed?
| Age Group | Number of Players |
| 6 – 7 Years old | 18 |
| 8 – 9 Years old | 24 |
Division equations:
Addition equations:

_____ trophies are needed
Answer:
7 trophies are needed.
Explanation:
As each age group is divided into teams with 6 players on each team and each team receives a trophy at the end of the season, so the number of trophies needed for age group 6-7 years old is 18÷6= 3 trophies. And for the age group 8-9 years old is 24÷6= 4 trophies.
Show and Grow
Question 8.
Each age group is put into cabins with 8 campers in each cabin. How many cabins are needed?
| Age Group | Number of Campers |
| 5 – 7 Years old | 32 |
| 8 – 10 Years old | 24 |
The two age groups are combined into one group. Does the total number of cabins that are needed change? Explain

Answer:
The number of cabins needed for the age group of 5-7 years is 4 cabins and the number of cabins needed for the age group of 8-10 years old is 3 cabins. There will be no change in the total number of cabins.
Explanation:
For the age group of 5-7 years old number of cabins needed are 32÷8= 4 cabins. And for the age group 8- 10 years old number of cabins needed are 24÷8= 3 cabins. If two age groups are combined into one group then the total number of campers are 24+32= 56. So the total number of cabins needed are 56÷8= 7 cabins. So there will be no change.
Use Number Lines to Divide Homework & Practice 1.7
Complete the equations
Question 1.
9 ÷ 3 = _____
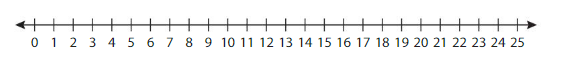
Answer:
9÷3= 3.
Explanation:
By using the number line, we will countback by 3s from 9 until we reach 0,
9÷3= 3, so there are 3 groups of 3.
Question 2.
20 – 5 = ____
____ – 5 = ____
____ – 5 = ____
____ – 5 = 0
____ ÷ ____ = ____
Answer:
20-5= 15,
15-5= 10,
10-5= 5,
5-5= 0,
20÷5= 4.
Explanation:
we will use repeated subtraction which starts from 20 as given and then we will subtract 5 until we get 0.
20-5= 15,
15-5= 10,
10-5= 5,
5-5= 0,
20÷5= 4.
Question 3.
10 – 2 = ____
____ – 2 = ____
____ – 2 = ____
____ – 2 = ____
____ – 2 = 0
____ ÷ ____ = ____
Answer:
10-2 = 8,
8-2= 6,
6-2= 4,
4-2= 2,
2-2= 0,
10÷5= 5.
Explanation:
we will use repeated subtraction which starts from 10 as given and then we will subtract 2 until we get 0.
10-2 = 8,
8-2= 6,
6-2= 4,
4-2= 2,
2-2= 0,
10÷5= 5.
Question 4.
YOU BE THE TEACHER
Descartes uses repeated subtraction to find 15 ÷ 5. Is he correct? Explain.
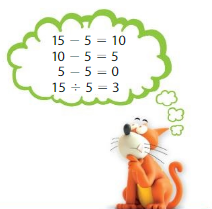
Answer: Yes, he is correct.
Explanation:
As Descartes uses repeated subtraction which starts from 15 as given and then we will subtract 5 until we get 0. So Descartes is correct.
Question 5.
DIG DEEPER!
Find Newton’s missing number. Explain how you solved.

Answer:
18-6= 12,
12-6= 6,
6-6 = 0.
Explanation:
Here, given subtract 6 from a number 3 times, and reach 0, so we will multiply 6 by 3 and we will get a number,
which is 6×3= 18. So the number is 18 if we subtract 6, 3 times we will reach 0.
18-6= 12,
12-6= 6,
6-6 = 0.
Question 6.
Modeling Real Life
Each age group is divided into groups of 7 swimmers. How many groups are there in each age group?
| Age Group | Number of Swimmers |
| 6 – 8 Years old | 28 |
| 9 – 11 Years old | 14 |
The two age groups are combined into one group. Does the total number of groups change? Explain.
Answer:
By combining two age groups into one group the total number of groups does not change, as the total number of groups and the combined two age group is equal.
Explanation:
As each age was divided into groups of 7 swimmers, so the number of groups in the 6-8 years old age group is 28÷7= 4 groups. And the number of groups in the 9-11 years old age group is 14÷7= 2 groups. And the total number of groups is 4+2= 6. As the two age groups are combined into one group, so 28+14= 42. So the total number of groups will be 42÷7= 6 groups.
Review & Refresh
Question 7.
Circle the values of the underlined digit.

Answer:
8 tens.
Explanation:
The value of 8 in the digit 581 is 8 tens.
Understand Multiplication and Division Performance Task
Question 1.
a. Your science teacher gives you 71 picture cards to sort into categories, living and nonliving. You sort 11 cards into the nonliving category. How many cards do you sort into the living category?

b. You sort the living cards into 2 categories, plants, and animals. The numbers of cards in each category are equal. How many cards are in the animal category?
c. You divide the animal cards into 6 equal groups. How many animal cards are in each group?
d. The animals in 5 of the groups have backbones, and the animals in the other group do not have backbones. How many more cards have animals with backbones? Explain.

Answer:
a) 60.
b) 30 cards in the animal category.
c) 5 animal cards.
d)
Explanation:
a) As there are 71 picture cards and 11 of them are sorted into the non-living category, so the remaining cards in the living category are 71-11= 60.
b) As the living cards are 60 and those are divided into two equal categories 60÷2= 30, which is 30 plants and 30 animal cards. So there are 30 cards in the animal category.
c) To divide the animal cards into 6 equal groups, we will divide 30 by 6 and there will be 30÷6= 5 animal cards are in each group.
d)
Understand Multiplication and Division Activity
Hooray Array!
Getting Started: Fill in your board with each number from the Number List. You may write each number in any square. Each square can only have one number.
Directions:
1. Choose a player to be the caller. The caller selects a Hooray Array Equation Card and reads the equation.
2. All players solve the equation and place a counter on the answer. Cover only 1 number per turn.
3. Repeat the process with players taking turns as the caller.
4. The winner is the first player who creates a 3 × 3 array on the board and yells, “HOORAY ARRAY!”
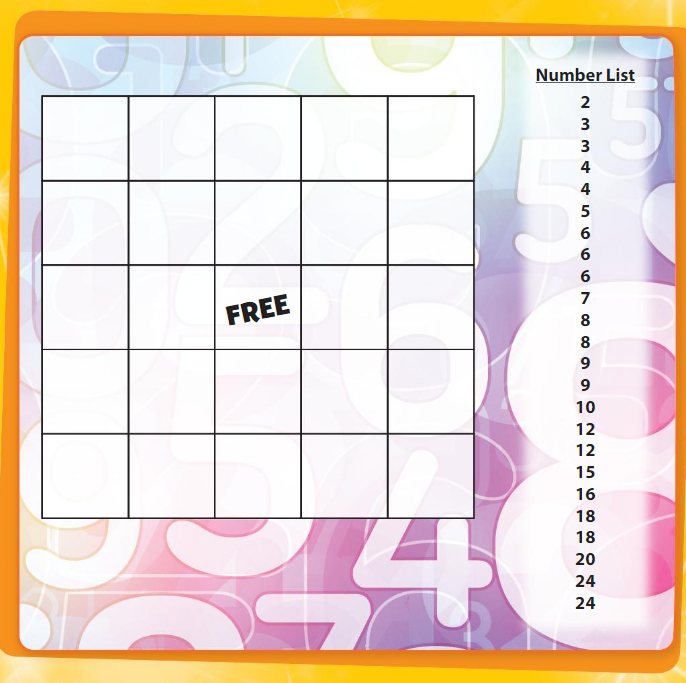
Answer:
Understand Multiplication and Division Chapter practice
1.1 Use Equal Groups to Multiply
Question 1.
Use the model to complete the statements.

Answer:
2 groups of 3,
3+3= 6,
2×3= 6.
Explanation:
Here, we can see 2 groups with 3 counters in each group.
So, 2 groups of 3 which is 6, which means 2×3= 6.
And if we add 2 groups of the counter, we will get the same result,
which is 3+3= 6.
Draw equal groups. Then complete the equations.
Question 2.
3 groups of 6
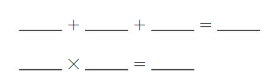
Answer:
3 groups of 6,
2+2+2= 6,
3×6= 18.
Explanation:
Here, we can see 3 groups with 6 counters in each group.
So, 3 groups of 6 which are 18, which means 3×6= 18.
And if we add 3 groups of the counter, we will get the same result,
which is 2+2+2= 6.
Question 3.
4 groups of 5
Answer:
4 groups of 5,
5+5+5+5= 20,
4×5= 20.
Explanation:
Here, we can see 4 groups with 5 counters in each group.
So, 4 groups of 5 which is 20, which means 4×5= 20.
And if we add 4 groups of the counter, we will get the same result,
which is 5+5+5+5= 20.
1.2 Use Number Lines to Multiply
Question 4.
Find 8 × 3
Number of jumps: ___
Size of each jump: ___
8 × 3 = ____
Answer:
8×3= 24.
Explanation:
Here, we will start at 0 and then we will skip count by 3s eight times. So, the number of jumps is 8, and the size of each jump is 3. which is 8×3= 24.
1.3 Use Arrays to Multiply
Draw an array to multiply.
Question 5.
2 × 8 = ___
Answer:
2 × 8 = 16.
Explanation:
To draw an array of 2×8, we will take 2 rows and 8 columns to build an array.

Question 6.
7 × 4 = ___
Answer:
7 × 4 = 28
Explanation:
To draw an array of 7×4, we will take 7 rows and 4 columns to build an array.

Question 7.
YOU BE THE TEACHER
Newton has 32 counters. He says that he can use all the counters to make an array with 6 rows. Is he correct? Explain.

Answer: No, Newton is not correct.
Explanation:
No, as Newton has 32 counters which will not make an array with 6 rows. As 32 is not divisible 6, so he is not correct.
1.4 Multiply in Any Order
Question 8.
Draw an array to show the Commutative Property of Multiplication. Complete the statements.

____ × ____ = ____
____ × ____ = ____
So, ___ × ___ = ____ × ___
Answer:
9×5 = 45,
5×9 = 45,
So, 9×5 = 5×9.
Explanation:
In the above image, we can see 9 rows of 5,
which means 9×5 = 45,
so by the commutative property of multiplication,
9×5 = 5×9,
which is 45.
Complete the equation.
Question 9.
4 × 10 = 10 × ___
Answer: 4.
Explanation:
By the commutative property of multiplication, which means changing the order of factors which does not change the product. So,
4 × 10 = 10 × 4.
Question 10.
3 × 9 = ____ × 3
Answer: 9
Explanation:
By the commutative property of multiplication, which means changing the order of factors which does not change the product. So,
3 × 9 = 9 × 3.
Question 11.
8 × ___ = 4 × 8.
Answer: 4
Explanation:
By the commutative property of multiplication, which means changing the order of factors which does not change the product. So,
8 × 4= 4 × 8.
1.5 Divide: Size of Equal Groups
Question 12.
Divide 27 counters into 3 equal groups. How many counters are in each group?

Use the tape diagram to model the equation.
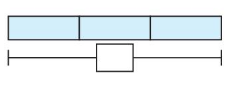
27 ÷ 3 = ___
Answer: 9 counters in each group.
Explanation:
Given Counters is 27,
now, we will divide 27 counters into groups of 3,
which means
27÷3= 9.
On dividing 27 counters by 3,
we will get the result as 9 groups.
Question 13
Divide 32 counters into4 equal groups. How many counters are in each group?
32 ÷ 4 = ___
Answer: 8 counters in each group.
Explanation:
Given Counters is 32,
now, we will divide 32 counters into groups of 4,
which means
32÷4= 8.
On dividing 32 counters by 4,
we will get the result as 8 counters in each group.
Question 14.
Divide 12 counters into4 equal groups. How many counters are in each group?
12 ÷ 4 = ___
Answer: 3 counters in each group.
Explanation:
Given Counters is 12,
now, we will divide 12 counters into groups of 4,
which means
12÷4= 3.
On dividing 12 counters by 4,
we will get the result as 3 counters in each group.
1.6 Divide: Number of Equal Groups
Question 15.
Divide 15 counters into groups of 3. How many groups are there?
15 ÷ 3 = ___
Answer: 5 groups.
Explanation:
Given Counters is 15,
now, we will divide 15 counters into groups of 3
which means
15÷3= 5.
On dividing 15 counters by 3,
we will get the result in 5 groups.
Question 16.
Divide 20 counters into groups of 2. How many groups are there?
20 ÷ 2 = __
Answer: 10 groups.
Explanation:
Given Counters is 20,
now, we will divide 20 counters into groups of 2,
which means
20÷2= 10.
On dividing 20 counters by 2,
we will get the result as 10 groups.
Question 17.
Modeling Real Life
Newton and Descartes are trying a new music app. Newton uses 4 credits a day to hear songs without commercials. Descartes uses 2 credits a day to hear songs with commercials. Who runs out of credits first? Explain.
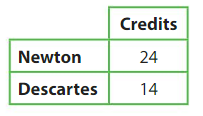
![]()
Answer:
Newton will run out of credits first than Descartes. No, they will not run out of credits at the same time.
Explanation:
The total credits do Newton has in a new music app are 24 and he uses 4 credits a day to hear songs without commercials, so the remaining credits are 24-4= 20. And the total credits Descartes had are 14 and he uses 2 credits a day to hear songs with commercials, which is 14-2= 12. we will use repeated subtraction which starts from 24 as given and then we will subtract 4 until we get 0 which is
24-4= 20
20-4= 16
16-4= 12
12-4= 8
8-4= 4
4-4= 0
so, Newton will run out of credits by his 6 uses. And we will use repeated subtraction for Descartes’s credits, which is
14-2= 12
12-2= 10
10-2= 8
8-2= 6
6-2= 4
4-2= 2
2-2= 0
so, Descartes will run out of credits by his 7 uses. So Newton will run out of credits earlier than Descartes.
1.7 Use Number Lines to Divide
Complete the equations.
Question 18.
28 ÷ 7 = ___
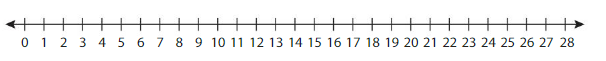
Answer:
28 ÷ 7 = 4.
Explanation:
By using the number line, we will countback by 7s from 28 until we reach 0,
28÷7= 4, so there are 4 groups of 7.
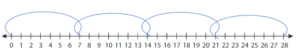
Question 19.
18 – 9 = ___
___ – 9 = 0
___ ÷ ___ = ___
Answer:
18-9= 0,
9-9= 0
18÷9= 2
Explanation:
We will use repeated subtraction which starts with 18 as given and then we will subtract 9 until we get 0. So the number of jumps is 2 and the size of the jump is 9.
Question 20.
12 – 4 = ___
___ – 4 = ___
___ – 4 = 0
___ ÷ ___ = ___
Answer:
12-4= 8
8-4= 4
4-4= 0
12÷4= 3.
Explanation:
We will use repeated subtraction which starts with 12 as given and then we will subtract 4 until we get 0. So the number of jumps is 3 and the size of the jump is 4.
Final Words:
The solutions seen in this chapter are as per the latest edition. The Big Ideas Math Book Grade 3 Chapter 1 Understand Multiplication and Division Answers are prepared by the math experts so you don’t worry about the solutions. I Hope the details prevailed in the Big Ideas Math Answers Grade 3 Chapter 1 Understand Multiplication and Division made you happy. Please share the Big Ideas Math Answer Key 3rd Grade 1st Chapter Understand Multiplication and Division with your friends and help them to overcome their difficulties in this chapter. Bookmark our site to get the solutions of all grade 3 chapters.
