The ratio indicates how many times a number contains another. Ratios are represented as fractions i.e a: b. The comparison or the simplified form of two quantities of the same kind is called the ratio. Interested students who want to know more about the concept of ratios can read this complete page. Here, we will discuss the basic concept of the ratio, key points, definition, and example questions.
Ratios – Definition
Ratios are an important concept in mathematics. In certain cases, the comparison of two quantities using the division method is difficult. So, at that time, we use ratio. The ratio gives us how many times one quantity is equal to another quantity.
Simply, a ratio is a number that is used to express one quantity as a fraction of another one. Two numbers in a ratio can be expressed only when they have the same unit. The sign of ratio is ‘:’. The real-life examples of a ratio are the rate of speed (distance/time), price of a material (rupees/meter, and others.
Key Points to Remember regarding Ratios
The key points to remember regarding the ratios are as follows:
- A ratio must exist between two quantities of the same kind
- To compare two things, their units should be the same
- There should be significant order of terms
- The comparison of two ratios can be performed, if the ratios are equivalent like fractions
Ratio Formulas
1. If we have two entities and you need to find the ratio of these two then the formula is defined as a: b or a/b.
Where a, b will be the entities
a is called the first term or antecedent and b is called the second term or consequent
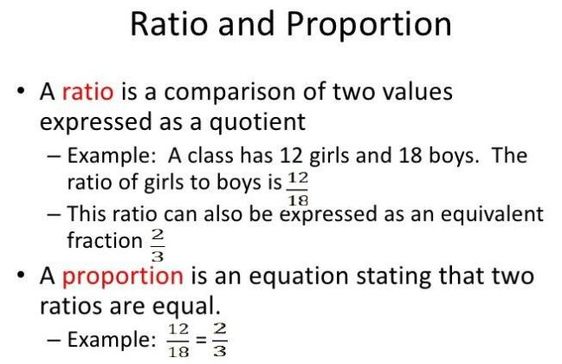
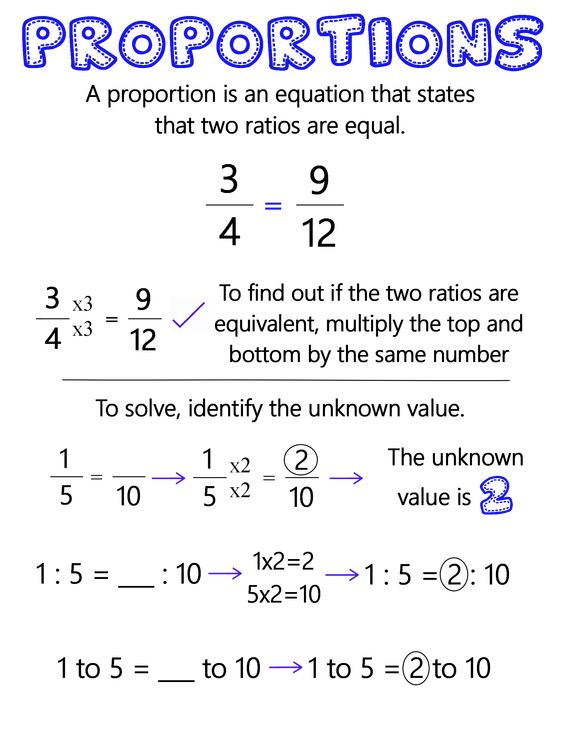
2. If two ratios are equal, then they are proportional
a : b = c : d
d is called the fourth proportional to a, b, c
c is called third proportion to a, b
The mean proportion between a and b is √(ab)
3. If (a : b) > (c : d) = (a/b > c/d)
The compounded ratio of the ratios (a : b), (c : d), (e : f) is (ace : bdf)
4. If a: b is a ratio, then
a²: b² is a duplicate ratio
√a: √b is a sub-duplicate ratio
a³: b³ is a triplicate ratio
5. Ratio and Proportion Tricks
If a/b = x/y, then ay = bx or a/x = b/y or b/a = y/x
If a/b = x/y, then \(\frac { a + b }{ b } =\frac { x + y }{ y } \) or \(\frac { a – b }{ b } =\frac { x – y }{ y } \)
If a/b = x/y, then \(\frac { a + b }{ a – b } =\frac { x + y }{ x – y } \) this is componendo dividendo rule
Also, Read
- What is Ratio and Proportion?
- Practice Test on Ratio and Proportion
- Worked out Problems on Ratio and Proportion
Solved Examples on Ratios
Example 1:
If x : y = 4 : 7, then find (4x – y) : (2x + 3y).
Solution:
Given ratio is x : y = 4 : 7
x = 4k, y = 7k
(4x – y) : (2x + 3y) = \(\frac { (4x – y) }{ (2x + 3y) } \) = \(\frac { (4 • 4k – 7k) }{ (2 • 4k + 3 • 7k) } \)
= \(\frac { (16k – 7k) }{ (8k + 21k) } \) = \(\frac { 9k }{ 29k } \)
= \(\frac { 9 }{ 29 } \)
= 9 : 29
Therefore, (4x – y) : (2x + 3y) = 9 : 29.
Example 2:
If a : b = 4 : 5, b : c = 15 : 8 then find a : c.
Solution:
Given that,
a : b = 4 : 5, b : c = 15 : 8
a : b = 4 : 5 = \(\frac { 4 }{ 5 } \) —– (i)
b : c = 15 : 8 = \(\frac { 15 }{ 8 } \) —– (ii)
By multiplying (i) and (ii), we get
\(\frac { a }{ b } \) x \(\frac { b }{ c } \) = \(\frac { 4 }{ 5 } \) x \(\frac { 15 }{ 8 } \)
\(\frac { a }{ c } \) = \(\frac { 3 }{ 2 } \)
Therefore, a : c = 3 : 2
Example 3:
If a quantity is divided in the ratio of 5: 7, the larger part is 84. Find the quantity.
Solution:
Given that,
A quantity is divided in the ratio of 5: 7
Let the quantity be x
Then the two quantities are \(\frac { 5x }{ 5 + 7 } \), \(\frac { 7x }{ 5 + 7 } \)
The larger part is 84
So, \(\frac { 7x }{ 5 + 7 } \) = 84
\(\frac { 7x }{ 12 } \) = 84
7x = 84 • 12
7x = 1008
x = \(\frac { 1008 }{ 7 } \)
x = 144
Therefore, the quantity is 144.
Example 4:
If (3a + 5b) : (7a – 4b) = 7 : 4 then find the ratio a : b.
Solution:
Given that,
(3a + 5b) : (7a – 4b) = 7 : 4
\(\frac { 3a + 5b }{ 7a – 4b } \) = \(\frac { 7 }{ 4 } \)
4(3a + 5b) = 7(7a – 4b)
12a + 20b = 49a – 28 b
20b + 28b = 49a – 12a
48b = 37a
\(\frac { 48 }{ 37 } \) = \(\frac { a }{ b } \)
So, a : b = 48 : 37

Frequently Asked Questions on Ratios
1. What are the different types of ratios?
The different types of ratios are compounded ratio, duplicate ratio, triplicate ratio, subtriplicate ratio, subduplicate ratio, the ratio of equalities, reciprocal ratio, the ratio of inequalities, the ratio of greater inequalities, and the ratio of lesser inequalities.
2. What are the 3 ways of writing ratios?
The three most used ways to write a ratio are given here. The first one is fraction 2/5. The second method is using a word to i.e 2 to 5. Finally, the third one is using the ratio colon between two numbers, 2: 5.
3. Define ratio with an example?
The ratio is a mathematical expression represented in the form of a: b, where a and b are two integers. It can also be expressed as a fraction. It is used to compare things or quantities. The example is 3: 4 = 3/4.
4. Write the differences between ratio and proportion?
The ratio is helpful to compare two things of the same unit whereas proportion is used to express the relation between two ratios. The ratio is represented using a colon: or slash / and proportion is represented using a double colon:: or equal to symbol =. The keyword to identify a ratio is “to every” and the proportion is “out of”.
